Advertisements
Advertisements
प्रश्न
In the following figure, BA || ED and BC || EF. Show that ∠ABC = ∠DEF
[Hint: Produce DE to intersect BC at P (say)].
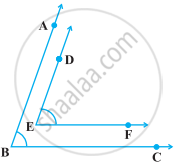
उत्तर
According to the question:
Given: Producing DE to intersect BC at P.
EF || BC and DP is the transversal,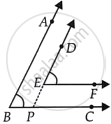
∠DEF = ∠DPC ...(i) [Corresponding ∠s]
See the above figure, AB || DP and BC is the transversal,
∠DPC = ∠ABC ...(ii) [Corresponding ∠s]
Now, from equation (i) and (ii), we get:
∠ABC = ∠DEF
Hence proved.
APPEARS IN
संबंधित प्रश्न
If two straight lines are perpendicular to the same line, prove that they are parallel to each
other.
Prove that the straight lines perpendicular to the same straight line are parallel to one
another.
If two interior angles on the same side of a transversal intersecting two parallel lines are in the ratio 2:3, then the measure of the larger angle is
In the given figure, PQ || RS, ∠AEF = 95°, ∠BHS = 110° and ∠ABC = x°. Then the value of x is

In the given figure, if l1 || l2, what is the value of y?

In the given figure, if AB || CD, then x =

In the given figure, If line segment AB is parallel to the line segment CD, what is the value of y?

Two lines l and m are perpendicular to the same line n. Are l and m perpendicular to each other? Give reason for your answer.
In the following figure, bisectors AP and BQ of the alternate interior angles are parallel, then show that l ||m.
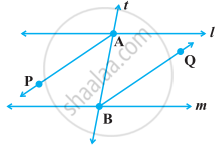
A transversal intersects two parallel lines. Prove that the bisectors of any pair of corresponding angles so formed are parallel.
