Advertisements
Advertisements
प्रश्न
In the following figure, find the value of x for which the lines l and m are parallel.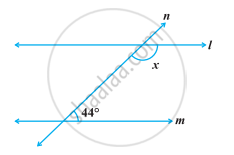
उत्तर
Let us draw the figure as below -
It is given to us that l and m are parallel to each other.
Here, n is a transversal intersecting l and m which are parallel to each other.
Also, we have ∠pqm = 44° ...(i)
We have to find the value of x, i.e., ∠qpl
We know, if a transversal intersects two parallel lines then each pair of corresponding angles is equal.
Here, the transversal n intersects two parallel lines l and m. So, the following holds true for the corresponding angles.
∠pqm = ∠npl
⇒ ∠npl = 44° (From (i), we have ∠pqm = 44°) ...(ii)
Again, the linear pair axiom states that
If a ray stands on a line, then the sum of two adjacent angles so formed is 180°.
Here, we can see that l is a ray standing on the line n.
⇒ ∠npl + ∠lpq = 180° ...(By linear pair axiom)
⇒ 44° + ∠lpq = 180°
⇒ ∠lpq = 180° – 44°
⇒ ∠lpq = 136°
⇒ x = 136°
Thus, the value of x is equal to 136°.
APPEARS IN
संबंधित प्रश्न
In the below fig, l, m and n are parallel lines intersected by transversal p at X, Y and Z
respectively. Find ∠1, ∠2 and ∠3.

If two straight lines are perpendicular to the same line, prove that they are parallel to each
other.
Which pair of lines in the below fig, is parallel? Given reasons.

Fill in the blank in the following to make the statement true:
If two parallel lines are intersected by a transversal, then interior angles on the same
side of the transversal are _______
Fill in the blank :
If a transversal intersects a pair of lines in such a way that the sum of interior angles
on the same side of transversal is 180°, then the lines are _______.
In the given figure, if l1 || l2, what is x + y in terms of w and z?
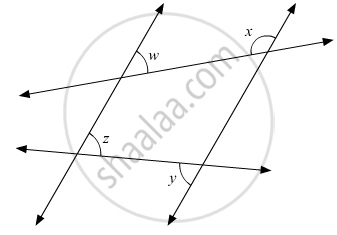
In the given figure, if l1 || l2 and l3 || l4, what is y in terms of x?

In the given figure, if lines l and m are parallel lines, then x =

In the given figure, if \[\frac{y}{x} = 5\] and \[\frac{z}{x} = 4\] then the value of x isc
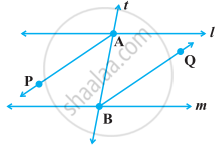
AP and BQ are the bisectors of the two alternate interior angles formed by the intersection of a transversal t with parallel lines l and m (Figure). Show that AP || BQ.