Advertisements
Advertisements
Question
In the following figure, find the value of x for which the lines l and m are parallel.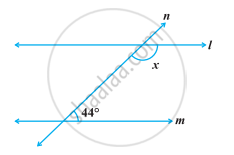
Solution
Let us draw the figure as below -
It is given to us that l and m are parallel to each other.
Here, n is a transversal intersecting l and m which are parallel to each other.
Also, we have ∠pqm = 44° ...(i)
We have to find the value of x, i.e., ∠qpl
We know, if a transversal intersects two parallel lines then each pair of corresponding angles is equal.
Here, the transversal n intersects two parallel lines l and m. So, the following holds true for the corresponding angles.
∠pqm = ∠npl
⇒ ∠npl = 44° (From (i), we have ∠pqm = 44°) ...(ii)
Again, the linear pair axiom states that
If a ray stands on a line, then the sum of two adjacent angles so formed is 180°.
Here, we can see that l is a ray standing on the line n.
⇒ ∠npl + ∠lpq = 180° ...(By linear pair axiom)
⇒ 44° + ∠lpq = 180°
⇒ ∠lpq = 180° – 44°
⇒ ∠lpq = 136°
⇒ x = 136°
Thus, the value of x is equal to 136°.
APPEARS IN
RELATED QUESTIONS
If two straight lines are perpendicular to the same line, prove that they are parallel to each
other.
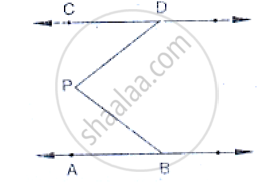
In the below fig, lines AB and CD are parallel and P is any point as shown in the figure.
Show that` ∠`ABP +` ∠`CDP = ∠DPB.
Prove that the straight lines perpendicular to the same straight line are parallel to one
another.
Which pair of lines in the below fig, is parallel? Given reasons.

Which of the following statement are true and false ? Give reason.
If two parallel lines are intersected by a transversal, then the interior angles on the same side of the transversal are equal.
In the given figure, if l1 || l2, what is the value of y?

In the given figure, if AB || CD, then x =

In the given figure, if lines l and m are parallel, then the value of x is

In the given figure, If line segment AB is parallel to the line segment CD, what is the value of y?

In the following figure, BA || ED and BC || EF. Show that ∠ABC = ∠DEF
[Hint: Produce DE to intersect BC at P (say)].