Advertisements
Advertisements
प्रश्न
In the given figure, PQ = PR, RS = RQ and ST || QR. If the exterior angle RPU is 140°, then the measure of angle TSR is ______.
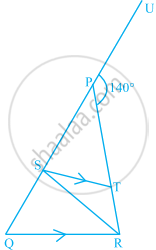
पर्याय
55°
40°
50°
45°
उत्तर
In the given figure, PQ = PR, RS = RQ and ST || QR. If the exterior angle RPU is 140°, then the measure of angle TSR is 40°.
Explanation:
Here,
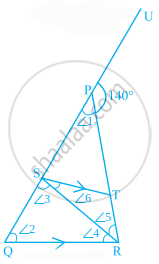
∠1 + ∠P = 180° ......[Linear pair]
⇒ ∠1 + 140° = 180°
⇒ ∠1 = 180° – 140°
⇒ ∠1 = 40°
Since, PQ = PR
∴ ∠Q = ∠R = x ......[Say]
In ΔPQR, ∠P + ∠Q + ∠R = 180° ......[Angle sum property of a triangle]
⇒ 40° + x + x = 180°
⇒ 2x = 180° – 40°
⇒ 2x = 140°
⇒ x = 70°
So, ∠Q = ∠R = 70°
Given that, RS = RQ
∴ ∠2 = ∠3 = 70°
In ΔSQR, ∠2 + ∠3 + ∠4 = 180° ......[Angle sum property of a triangle]
⇒ 70° + 70° + ∠4° = 180°
⇒ ∠4 = 180° – 140°
⇒ ∠4 = 40°
Also, ST || QR ......[Given]
Now, ∠4 = ∠6 = 40° ......[Alternate interior angles]
∴ ∠TSR = 40°
APPEARS IN
संबंधित प्रश्न
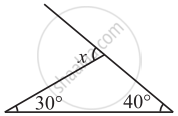
Find the value of the unknown exterior angle x in the following diagram:
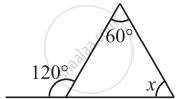
Find the value of the unknown interior angle x in the following figure.
Using the measures of the angles given in the figure alongside, find the measures of the remaining three angles.

In the isosceles triangle ABC, ∠A, and ∠B are equal. ∠ACD is an exterior angle of ∆ABC. The measures of ∠ACB and ∠ACD are (3x − 17)° and (8x + 10)°, respectively. Find the measures of ∠ACB and ∠ACD. Also find the measures of ∠A and ∠B.
Find angle x in Fig

Find the value of x in the given triangle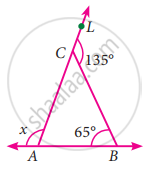
Using the diagram find the value of x.
In ∆PQR, if ∠P = 60°, and ∠Q = 40°, then the exterior angle formed by producing QR is equal to ______.
In the given figure, ∠PRS = ∠ ______ + ∠ _______
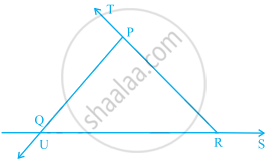
In the given figure, ∆PQR is right-angled at P. U and T are the points on line QRF. If OP || ST and US || RP, find ∠S.

