Advertisements
Advertisements
प्रश्न
In the isosceles triangle ABC, ∠A, and ∠B are equal. ∠ACD is an exterior angle of ∆ABC. The measures of ∠ACB and ∠ACD are (3x − 17)° and (8x + 10)°, respectively. Find the measures of ∠ACB and ∠ACD. Also find the measures of ∠A and ∠B.
उत्तर
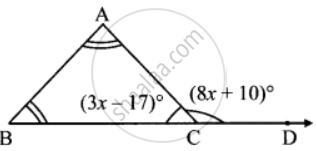
Given:
∠ACB = (3x − 17)∘
∠ACD = (8x + 10)∘
Now, ∠ACB + ∠ACD = 180∘ ...(Linear Pair angles)
⇒ 3x° − 17° + 8x° + 10° = 180°
⇒ 3x° + 8x° − 17° + 10° = 180°
⇒ 11x° − 7° = 180°
⇒ 11x° – 7° + 7° = 180° + 7 ...(Adding 7 on both sides.)
⇒ 11x° = 187°
⇒ x° = `187°/11°`
⇒ x° = 17°
Therefore,
∠ACB = (3x − 17)°
= (3 × 17)° − 17°
= (51 − 17)°
= 34°
∠ACD = (8x + 10)°
= (8 × 17)° + 10°
= (136 + 10)°
= 146°
Now, ∠A + ∠B = ∠ACD ...(Exterior angle property)
⇒ 2∠A = 146° (∵∠A = ∠B)
⇒ ∠A = `146°/2`
⇒ ∠A = 73°
Hence, the measures of ∠ACB, ∠ACD, ∠A and ∠B are 146°, 34°, 73° and 73°, respectively.
संबंधित प्रश्न
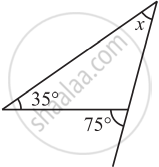
Find the value of the unknown interior angle x in the following figure.
Using the measures of the angles given in the figure alongside, find the measures of the remaining three angles.

In ∆LMN, MN is extended to O. If ∠MLN = 100 – x, ∠LMN = 2x and ∠LNO = 6x – 5, find the value of x
If an exterior angle of a triangle is 115° and one of the interior opposite angles is 35°, then the other two angles of the triangle are
In the given figure BD = BC, find the value of x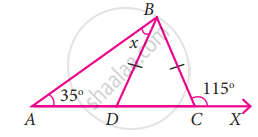
In the given figure, if AB || CD, then ______.
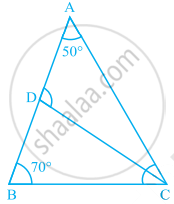
In ∆ABC, ∠Α = 50°, ∠B = 70° and bisector of ∠C meets AB in D (see figure). Measure of ∠ADC is ______.
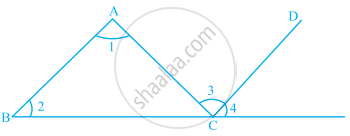
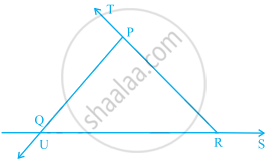
In the given figure, ∠TPQ = ∠ _____ + ∠ _____
In the given figure, ∠UQR = ∠______ + ∠ ______
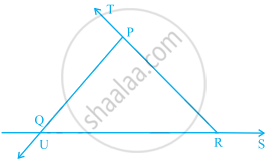
In the given figure, ∆PQR is right-angled at P. U and T are the points on line QRF. If OP || ST and US || RP, find ∠S.

