Advertisements
Advertisements
प्रश्न
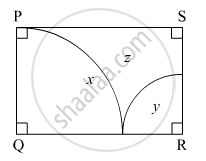
In the given figure,
\[\square\] PQRS is a rectangle. If PQ = 14 cm, QR = 21 cm, find the areas of the parts x, y, and z.
उत्तर
PQRS is a rectangle.
∴ ∠Q = ∠R = 90º

Radius of sector PTQ = PQ = 14 cm
∴ Area of part x = Area of the sector PTQ = \[\frac{\theta}{360° } \times \pi r^2 = \frac{\angle Q}{360° } \times \pi \times \left( PQ \right)^2 = \frac{90° }{360° } \times \frac{22}{7} \times \left( 14 \right)^2\] = 154 cm2
Radius of sector TUR = TR = QR − QT = QR − PQ = 21 − 14 = 7 cm (QT = PQ)
∴ Area of part y = Area of the sector TUR = \[\frac{\theta}{360° } \times \pi r^2 = \frac{\angle R}{360° } \times \pi \times \left( TR \right)^2 = \frac{90°}{360° } \times \frac{22}{7} \times \left( 7 \right)^2\] = 38.5 cm2
Now,
Area of rectangle PQRS = QR × PQ = 21 × 14 = 294 cm2
∴ Area of part z = Area of rectangle PQRS − Area of part x − Area of part y = 294 − 154 − 38.5 = 101.5 cm2
संबंधित प्रश्न
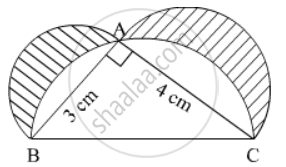
In the given figure, ∆ ABC is a right-angled triangle in which ∠ A is 90°. Semicircles are drawn on AB, AC and BC as diameters. Find the area of the shaded region
How many times a wheel of radius 28 cm must rotate to go 352 m? (Take `pi = 22/7`)
A horse is tied to a pole with 28m long string. Find the area where the horse can graze.
In circle of radius 6cm, chord of length 10 cm makes an angle of 110° at the centre of circle find Length of arc
The difference between the circumference and radius of a circle is 37cm. Using `pi = 22/7` , find the circumference of the circle.
Find the area of a quadrant of a circle whose circumference is 44 cm.
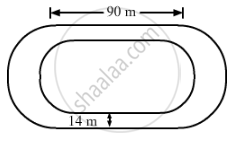
The inside perimeter of a running track shown in the figure is 400 m. The length of each of the straight portions is 90 m, and the ends are semicircles. If the track is 14 m wide everywhere, find the area of the track. Also, find the length of the outer boundary of the track.
The perimeter of a sector of a circle of radius 5.6 cm is 27.2 cm. Find the area of the sector.
Find the area of the circle if its circumfence is 88 cm.
Find the area of a circular field that has a circumference of 396 m.
The base circumferences of two cones are the same. If their slant heights are in the ratio 5 : 4, find the ratio of their curved surface areas.
A cylindrical beaker of 7 cm diameter is partly filled with water. Determine the number of spherical marbles of diameter 1.4 cm that are to be submerged in it to raise the water level by 5.6 cm
The wheel of a bullock cart has a diameter of 1.4 m. How many rotations will the wheel complete as the cart travels 1.1 km?
Find the area and perimeter of the circles with following: Diameter = 77cm
In a circle of diameter 42 cm, if an arc subtends an angle of 60° at the centre, where `pi = 22/7` then length of an arc is ____________.
Is it true that the distance travelled by a circular wheel of diameter d cm in one revolution is 2 π d cm? Why?
In reference to a circle the value of π is equal to ______.
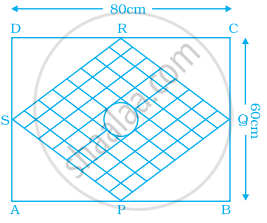
ABCD is a given rectangle with length as 80 cm and breadth as 60 cm. P, Q, R, S are the midpoints of sides AB, BC, CD, DA respectively. A circular rangoli of radius 10 cm is drawn at the centre as shown in the given figure. Find the area of shaded portion.