Advertisements
Advertisements
प्रश्न
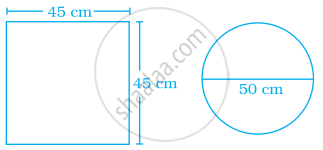
Pizza factory has come out with two kinds of pizzas. A square pizza of side 45 cm costs ₹ 150 and a circular pizza of diameter 50 cm costs ₹ 160 (Figure). Which pizza is a better deal?
उत्तर
Given, side of square pizza = 45 cm
∴ Area of a square pizza = (Side)2 = (45)2 = 2025 cm2
Diameter of circular pizza = 50 cm
∴ Radius = `50/2` = 25 cm ......`[∵ "Radius" = "Diameter"/2]`
Now, area of the circular pizza = `22/1 xx 25 xx 25`
= `22/7 xx 625`
= `13750/7`
= 1964.28 cm2 ......[∵ Area of circle = πr2]
∴ Price of 1 cm square pizza = `2025/150` = ₹ 13.5
and price of 1 cm circular pizza = `1964.28/160` = ₹ 12.27
Hence, the circular pizza is a better deal.
APPEARS IN
संबंधित प्रश्न
A circle of radius 2 cm is cut out from a square piece of an aluminium sheet of side 6 cm. What is the area of the left over aluminium sheet? (Take π = 3.14)
From a circular piece of cardboard of radius 3 cm two sectors of 900 have been cutoff . Find the perimeter of the remaining portion nearest hundredth centimeters ( `"Take" pi = 22/ 7`).
Find the area of minor segment of a circle of radius 14 cm, when the angle of the corresponding sector is 600 .
The diameter of a coin is 1 cm (in the following figure). If four such coins be placed on a table so that the rim of each touches that of the other two, find the area of the shaded region (Take π = 3.1416).
Write the formula for the area of a segment in a circle of radius r given that the sector angle is \[\theta\] (in degrees).
If a wire is bent into the shape of a square, then the area of the square is 81 cm2. When wire is bent into a semi-circular shape, then the area of the semi-circle will be ______.
The diameter of the wheels of a bus is 140 cm. How many revolutions per minute do the wheels make when the bus is moving at a speed of 72.6 km per hour?
If three circles of radius a each, are drawn such that each touches the other two, prove that the area included between them is equal to `4/25"a"^2`.
Find the area of a circle of radius 30 cm (use π = 3.14).
Find the area of the dining table whose diameter is 105 cm
