Advertisements
Advertisements
प्रश्न
If a wire is bent into the shape of a square, then the area of the square is 81 cm2. When wire is bent into a semi-circular shape, then the area of the semi-circle will be ______.
पर्याय
22 cm2
44 cm2
77 cm2
154 cm2
उत्तर
If a wire is bent into the shape of a square, then the area of the square is 81 cm2. When wire is bent into a semi-circular shape, then the area of the semi-circle will be 77 cm2.
Explanation:
We have given that a wire is bent in the form of square of side a cm such that the area of the square is `81 cm^2`. If we bent the same wire in the form of a semicircle with radius r cm, the perimeter of the wire will not change.
∴ perimeter of the square = perimeter of semi circle
`4a=1/2 (2pir)+2r` ...(1)
We know that area of the square = 81 cm2
∴ a2 = 81
∴ a = 9
Now we will substitute the value of a in the equation (1),
`4xx9=1/2(2pi r)+2r`
`∴ 36=1/2(2pir)+2r`
`∴36=(pir)+2r`
`∴36=r(pi+2)`
Now we will substitute `pi=22/7`
`∴36=r(22/7+2)`
`∴36=r((22+14)/7)`
`∴36=r(36/7)`
Multiplying both sides of the equation by 7 we get, 36 × 7 = r × 36
Now we will divide both sides of the equation by 36 we get, r = 7
Therefore, radius of the semi circle is 7cm.
Now we will find the area of the semicircle.
Area of the semicircle = `1/2xxpir^2`
= `1/2xxpixx7^2`
= `1/2xx22/7xx7^2`
= 11 × 7
= 77
Therefore, the area of the semicircle is 77 cm2.
APPEARS IN
संबंधित प्रश्न
A circular flower garden has an area of 314 m2. A sprinkler at the centre of the garden can cover an area that has a radius of 12 m. Will the sprinkler water the entire garden? (Take π = 3.14)
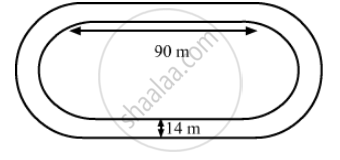
The inside perimeter of a running track (shown in the following figure) is 400 m. The length of each of the straight portion is 90 m and the ends are semi-circles. If the track is everywhere 14 m wide. find the area of the track. Also find the length of the outer running track.
In the following figure, the square ABCD is divided into five equal parts, all having same area. The central part is circular and the ines AE, GC, BF and HD lie along the diagonals AC and BD of the square. If AB = 22 cm, find:
the circumference of the central part.

The ratio of the areas of a circle and an equilateral triangle whose diameter and a side are respectively equal, is
If diameter of a circle is increased by 40%, then its area increase by
The area of a circle is 98.56 cm2. Find its circumference.
There is a path of uniform width 7 m round and outside a circular garden of diameter 210 m. Find the area of the path.
On a square cardboard sheet of area 784 cm2, four congruent circular plates of maximum size are placed such that each circular plate touches the other two plates and each side of the square sheet is tangent to two circular plates. Find the area of the square sheet not covered by the circular plates.
What is the diameter of a circle whose area is equal to the sum of the areas of two circles of radii 40cm and 9cm?
Read the following passage:
People of a circular village Dharamkot want to construct a road nearest to it. The road cannot pass through the village. But the people want the road at a shortest distance from the centre of the village. Suppose the road starts from A which is outside the circular village (as shown in the figure) and touch the boundary of the circular village at B such that AB = 20 m. Also the distance of the point A from the centre O of the village is 25 m. |
Based on the above information, answer the following questions:
- If B is the mid-point of AC, then find the distance AC.
- Find the shortest distance of the road from the centre of the village.
- Find the circumference of the village.
OR
Find the area of the village.


