Advertisements
Advertisements
प्रश्न
PQRS is a parallelogram. M and N are the mid-points of the adjacent sides QR and RS. O is the mid-point of the diagonal PR. Prove that MONR is a rectangle and MN is half of PR.
उत्तर

In ΔSRQ,
N and O are the mid-points of SR and PR respectively.
Therefore, ON || QR and ON = `(1)/(2)"QR"` i.e. ON = MR ........(i)
PR = SQ ...(diagonals of rectangle are equal and bisect each other)
⇒ O is mid-point of SQ
In ΔRQS,
M and O are the mid-points of QR and SQ respectively.
Therefore, OM || SR and OM = `(1)/(2)"QR"` i.e. OM = SQ ........(ii)
∠MRN = ∠QRS = 90° ...........(iii) (PQRS is a rectangle)
From (i) , (ii) and (iii)
Therefore, quadrilateral MONR has two opposite pairs of sides equal and parallel and an interior angle as right angle, so it is a rectangle.
In ΔSQR,
M and N are the mid-points of QR and SR respectively.
Therefore, MN || SQ and MN = `(1)/(2)"SQ"`
But SQ = PR
⇒ MN = `(1)/(2)"PR"`.
APPEARS IN
संबंधित प्रश्न
The diagonals of a rectangle intersect each other at right angles. Prove that the rectangle is a square.
Prove that the bisectors of interior angles of a parallelogram form a rectangle.
The diagonals PR and QS of a quadrilateral PQRS are perpendicular to each other. A, B, C and D are mid-point of PQ, QR, RS and SP respectively. Prove that ABCD is a rectangle.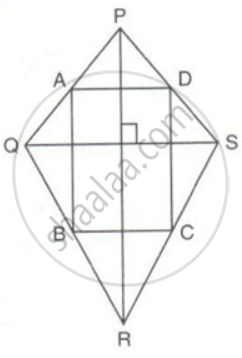
ABCD is a quadrilateral in which diagonals AC and BD intersect at a point O. Prove that: areaΔAOD + areaΔBOC + areaΔABO + areaΔCDO.
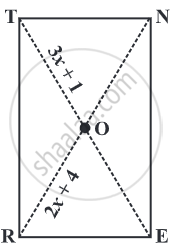
Length of one of the diagonals of a rectangle whose sides are 10 cm and 24 cm is ______.
Diagonals of a rectangle are ______.
Diagonals of a rectangle are equal.
Diagonals of rectangle bisect each other at right angles.
A photo frame is in the shape of a quadrilateral. With one diagonal longer than the other. Is it a rectangle? Why or why not?
