Advertisements
Advertisements
प्रश्न
Prove that the line segment joining the mid-points of the diagonals of a trapezium is parallel to each of the parallel sides, and is equal to half the difference of these sides.
उत्तर
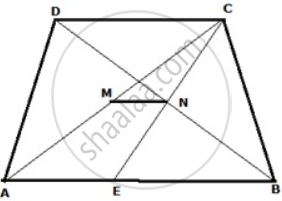
Join AC and BD. M and N are mid-points of AC and BD respectively. Join MN. Draw a line CN cutting AB at E.
Now, in Δs DBC and BNE,
DN = NB ...(N is the mid-point of BD, given)
∠CDB = ∠EBN ...(Alternate angles as DC || AB)
∠DNC = BNE ...(Vertically opposite angles)
⇒ ΔDNC ≅ ΔBNE ...(By A-S-A Test)
⇒ DC = BE
By Mid-point Theorem, in ΔACE, M and N are mid-points
MN = `(1)/(2)"AE" and "MN" || "AE" or "MN" || "AB"`
Also, AB || CD, therefore, MN || CD
⇒ MN = `(1)/(2)["AB" = "BE"]`
⇒ MN = `(1)/(2)["AB" = "CD"]` ...(since BE = CD)
⇒ MN = `(1)/(2)` x Difference of parallel sides AB and CD.
APPEARS IN
संबंधित प्रश्न
SN and QM are perpendiculars to the diagonal PR of parallelogram PQRS.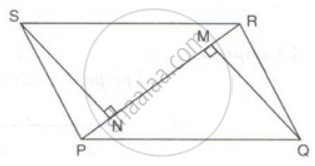
Prove that:
(i) ΔSNR ≅ ΔQMP
(ii) SN = QM
PQRS is a parallelogram. PQ is produced to T so that PQ = QT. Prove that PQ = QT. Prove that ST bisects QR.
ABCD is a rectangle with ∠ADB = 55°, calculate ∠ABD.
ABCD is a quadrilateral P, Q, R and S are the mid-points of AB, BC, CD and AD. Prove that PQRS is a parallelogram.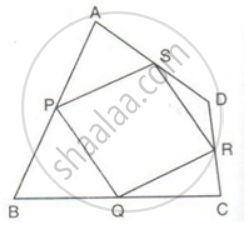
In a parallelogram PQRS, M and N are the midpoints of the opposite sides PQ and RS respectively. Prove that
RN and RM trisect QS.
In the given figure, PQRS is a trapezium in which PQ ‖ SR and PS = QR. Prove that: ∠PSR = ∠QRS and ∠SPQ = ∠RQP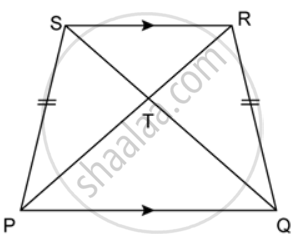
Prove that the diagonals of a parallelogram divide it into four triangles of equal area.
PQRS is a parallelogram and O is any point in its interior. Prove that: area(ΔPOQ) + area(ΔROS) - area(ΔQOR) + area(ΔSOP) = `(1)/(2)`area(|| gm PQRS)
In ΔABC, the mid-points of AB, BC and AC are P, Q and R respectively. Prove that BQRP is a parallelogram and that its area is half of ΔABC.
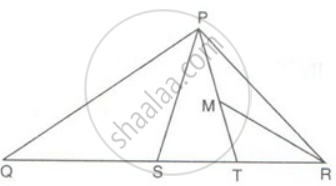
In ΔPQR, PS is a median. T is the mid-point of SR and M is the mid-point of PT. Prove that: ΔPMR = `(1)/(8)Δ"PQR"`.