Advertisements
Advertisements
प्रश्न
In ΔPQR, PS is a median. T is the mid-point of SR and M is the mid-point of PT. Prove that: ΔPMR = `(1)/(8)Δ"PQR"`.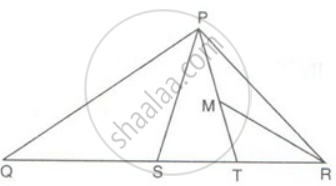
उत्तर
Area(ΔPQR) = area(ΔPQS) + area(ΔPSR) ....(i)
Since PS is the median of ΔPQR and median divides a triangle into two triangles of equal area.
Therefore, area(ΔPQS) = area(ΔPSR)
Substituting in (i)
Area(ΔPQR) = area(ΔPSR) + area(ΔPSR)
Area(ΔPQR) = 2area(ΔPSR) .........(iii)
Area(ΔPSR) = area(ΔPST) + area(ΔPTR) .....(iv)
Since PT is the median of ΔPSR and median divides a triangle into triangles of equal area.
Therefore, area(ΔPST) = area(ΔPTR) .....(v)
Substituting in (v)
Area(ΔPSR) = 2area(ΔPTR) ........(vi)
Substituting in (iii)
Area(ΔPQR) = 2 x 2area(ΔPTR)
Area(ΔPQR) = 4area(ΔPTR) .........(vii)
Area(ΔPQR) = area(ΔPMR) + area(ΔMTR) .....(viii)
Since MR is the median of ΔPTR and median divides a triangle into two triangles of equal area.
Therefore, area(ΔPMR) = area(ΔMTR) ....(ix)
Substituting in (viii)
Area(ΔPQR) = 4 x 2area(ΔPMR)
Area(ΔPQR) = 8 x area(ΔPMR)
area(ΔPMR) = `(1)/(8)"area(ΔPQR)"`.
APPEARS IN
संबंधित प्रश्न
SN and QM are perpendiculars to the diagonal PR of parallelogram PQRS.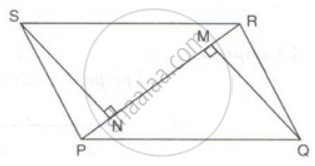
Prove that:
(i) ΔSNR ≅ ΔQMP
(ii) SN = QM
ABCD is a parallelogram. P and T are points on AB and DC respectively and AP = CT. Prove that PT and BD bisect each other.
PQRS is a parallelogram. T is the mid-point of RS and M is a point on the diagonal PR such that MR = `(1)/(4)"PR"`. TM is joined and extended to cut QR at N. Prove that QN = RN.
In a parallelogram PQRS, M and N are the midpoints of the opposite sides PQ and RS respectively. Prove that
PMRN is a parallelogram.
In the given figure, PQRS is a parallelogram in which PA = AB = Prove that: SA ‖ QB and SA = QB.
In the given figure, PQRS is a parallelogram in which PA = AB = Prove that: SAQB is a parallelogram.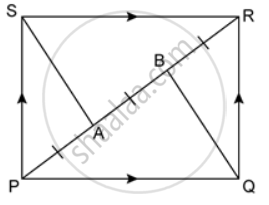
Prove that the diagonals of a parallelogram divide it into four triangles of equal area.
The diagonals AC and BC of a quadrilateral ABCD intersect at O. Prove that if BO = OD, then areas of ΔABC an ΔADC area equal.
In the given figure, AB ∥ SQ ∥ DC and AD ∥ PR ∥ BC. If the area of quadrilateral ABCD is 24 square units, find the area of quadrilateral PQRS.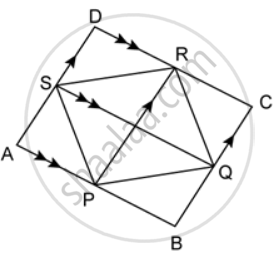
In ΔABC, the mid-points of AB, BC and AC are P, Q and R respectively. Prove that BQRP is a parallelogram and that its area is half of ΔABC.
