Advertisements
Advertisements
प्रश्न
Prove that the line segment joining the midpoints of two sides of a triangle is parallel to the third side whose length is half of the length of the third side
उत्तर
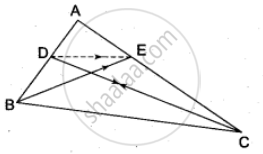
In ΔABC
`vec"OA" = vec"a"`
`vec"OB" = vec"b"` and
`vec"OC" = vec"c"`
D = midpoint of AB = `vec"OD" = (vec"a" + vec"b")/2`
E = midpoint of AB = `vec"OE" = (vec"a" + vec"c")/2`
Now `vec"DE" = vec"OE" - vec"D" = (vec"a" + vec"c")/2 - (vec"a" + vec"b")/2`
= `(vec"a" + vec"c" - vec"a" + vec"b")/2`
= `(vec"c" - vec"b")/2`
= `vec"BC"/2`
`vec"DE" = vec"BC"/2`
⇒ `vec"DE" || "to" vec"BC"` and half of BC.
APPEARS IN
संबंधित प्रश्न
Prove that the relation R defined on the set V of all vectors by `vec"a" "R" vec"b"` if `vec"a" = vec"b"` is an equivalence relation on V
Let `vec"a"` and `vec"b"` be the position vectors of the points A and B. Prove that the position vectors of the points which trisects the line segment AB are `(vec"a" + 2vec"b")/3` and `(vec"b" + 2vec"a")/3`
If D and E are the midpoints of the sides AB and AC of a triangle ABC, prove that `vec"BE" + vec"DC" = 3/2vec"BC"`
If `vec"PO" + vec"OQ" = vec"QO" + vec"OR"`, prove that the points P, Q, R are collinear
If G is the centroid of a triangle ABC, prove that `vec"GA" + vec"GB" + vec"GC" = vec0`
Let A, B, and C be the vertices of a triangle. Let D, E, and F be the midpoints of the sides BC, CA, and AB respectively. Show that `vec"AD" + vec"BE" + vec"CF" = vec0`
If ABCD is a quadrilateral and E and F are the midpoints of AC and BD respectively, then Prove that `vec"AB" + vec"AD" + vec"CB" + vec"CD" = 4vec"EF"`
The position vectors of the vertices of a triangle are `hat"i" + 2hat"j" + 3hat"k", 3hat"i" - 4hat"j" + 5hat"k"` and `-2hat"i" + 3hat"j" - 7hat"k"`. Find the perimeter of the triangle
Show that the points A(1, 1, 1), B(1, 2, 3) and C(2, – 1, 1) are vertices of an isosceles triangle
Choose the correct alternative:
The value of `vec"AB" + vec"BC" + vec"DA" + vec"CD"` is
Choose the correct alternative:
If `vec"BA" = 3hat"i" + 2hat"j" + hat"k"` and the position vector of is `hat"i" + 3hat"j" - hat"k"`, then the position vector A is
Choose the correct alternative:
If `vec"a", vec"b"` are the position vectors A and B, then which one of the following points whose position vector lies on AB, is
Choose the correct alternative:
If `vec"r" = (9vec"a" + 7vec"b")/16`, then the point P whose position vector `vec"r"` divides the line joining the points with position vectors `vec"a"` and `vec"b"` in the ratio
