Advertisements
Advertisements
प्रश्न
Solve the following quadratic equations by factorization:
3x2 = -11x - 10
उत्तर
We have been given
3x2 = -11x - 10
3x2 + 11x + 10 = 0
3x2 + 6x + 5x + 10 = 0
3x(x + 2) + 5(x + 2) = 0
(x + 2)(3x + 5) = 0
Therefore,
3x + 5 = 0
3x = -5
x = -5/3
or,
x + 2 = 0
x = -2
Hence, x = -5/3 or x = -2
APPEARS IN
संबंधित प्रश्न
Two number differ by 4 and their product is 192. Find the numbers?
A pole has to be erected at a point on the boundary of a circular park of diameter 13 meters in such a way that the difference of its distances from two diametrically opposite fixed gates A and B on the boundary is 7 meters. Is it the possible to do so? If yes, at what distances from the two gates should the pole be erected?
`3x^2-x-2=0`
Solve the following quadratic equation by factorisation.
`sqrt2 x^2 + 7x + 5sqrt2 = 0` to solve this quadratic equation by factorisation, complete the following activity.
`sqrt2 x^2 + 7x + 5sqrt2 = 0`
`sqrt2x^2+square+square+5sqrt2=0`
`x("______") + sqrt2 ("______") = 0`
(______) (x + 2) = 0
(______) = 0 or (x + 2) = 0
∴ x = `square` or x = - 2
∴ `square` and `sqrt(-2)` are roots of the equation.
Find the discriminant of the quadratic equation \[3\sqrt{3} x^2 + 10x + \sqrt{3} = 0\].
In a two digit number, the unit’s digit is twice the ten’s digit. If 27 is added to the number, the digit interchange their places. Find the number.
A person was given Rs. 3000 for a tour. If he extends his tour programme by 5 days, he must cut down his daily expenses by Rs. 20. Find the number of days of his tour programme.
Find the roots of the following quadratic equation by the factorisation method:
`3x^2 + 5sqrt(5)x - 10 = 0`
In the centre of a rectangular lawn of dimensions 50 m × 40 m, a rectangular pond has to be constructed so that the area of the grass surrounding the pond would be 1184 m2 [see figure]. Find the length and breadth of the pond.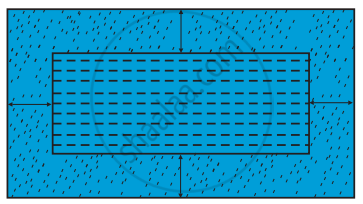
4x2 – 9 = 0 implies x is equal to ______.
