Advertisements
Advertisements
प्रश्न
The angles of depression of the top and bottom of a 50 m high building from the top of a tower are 45° and 60° respectively. Find the height of the tower and the horizontal distance between the tower and the building (use `sqrt3`=1.73)
उत्तर

Let the height of the tower AB be h m and the horizontal distance between the tower and the building BC be x m.
So,
AE=(h−50) m
In ∆AED,
`tan45^@=(AE)/(ED)`
`=>1=(h-50)/x`
⇒x = h−50 .....(1)
In ∆ABC,
`tan^@=(AB)/(BC)`
`=>sqrt3=h/x`
`=>x=sqrt3=h `
Using (1) and (2), we get
`x=sqrt3x-50`
`=>x=(sqrt3-1)=50`
`=>x=(50(sqrt3+1))/2=25xx2.73=68.25m`
Substituting the value of x in (1), we get
68.25 = h −50
⇒ h = 68.25 + 50
⇒h=118.25 m
Hence, the height of tower is 118.25 m and the horizontal distance between the tower and the building is 68.25 m.
APPEARS IN
संबंधित प्रश्न
A man standing on the deck of a ship, which is 10 m above water level, observes the angle of elevation of the top of a hill as 60° and the angle of depression of the base of a hill as 30°. Find the distance of the hill from the ship and the height of the hill
Two ships are there in the sea on either side of a light house in such a way that the ships and the light house are in the same straight line. The angles of depression of two ships as observed from the top of the light house are 60° and 45°. If the height of the light house is 200 m, find the distance between the two ships. [use √3=1.73]
If a vertical pole 6m high has a shadow of length 2 √3 metres, find the angle of elevation of the sun.
A 1.6 m tall girl stands at a distance of 3.2 m from a lamp-post and casts a shadow of 4.8 m on the ground. Find the height of the lamp-post by using (i) trigonometric ratios (ii) property of similar triangles.
The horizontal distance between two poles is 15 m. The angle of depression of the top of first pole as seen from the top of second pole is 30°. If the height of the second pole is 24 m, find the height of the first pole. Use`[sqrt3=1.732]`
Two poles of equal heights are standing opposite each other on either side of the road, which is 80 m wide. From a point between them on the road, the angels of elevation of the top of the poles are 60° and 30° respectively.
Find the height of the poles and the distances of the point from the poles.
A plane is observed to be approaching the airport. It is at a distance of 12 km from the point of observation and makes an angle of elevation of 60°. The height above the ground of the plane is ____________.
The angles of depression of the top and the bottom of a 10 m tall building from the top of a multi-storeyed building are 30° and 45°, respectively. Find the height of the multi-storeyed building.
From the top of a tower h m high, the angles of depression of two objects, which are in line with the foot of the tower are α and β (β > α). Find the distance between the two objects.
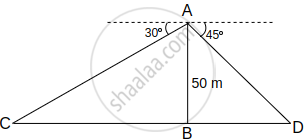
In the given figure, AB is tower of height 50 m. A man standing on its top, observes two car on the opposite sides of the tower with angles of depression 30° and 45° respectively. Find the distance between the two cars.