Advertisements
Advertisements
प्रश्न
The circumference of the base of the cylindrical vessel is 132 cm and its height is 25 cm. How many litres of water can it hold? (1000 cm3 = 1l)
`["Assume "pi=22/7]`
The circumference of the base of a cylindrical vessel is 132 cm and its height is 25 cm. Find the
1) radius of the cylinder
2) volume of the cylinder (Use `pi=22/7`)
उत्तर १
Let the radius of the cylindrical vessel be r.
Height (h) of vessel = 25 cm
Circumference of vessel = 132 cm
2πr = 132 cm
`r = (132xx7)/(2xx22)cm=21cm`
Volume of cylindrical vessel = πr2h
`=[22/7xx(21)^2xx25]cm^3`
= 34650 cm3
`=(34650/1000)litres" "[because" 1 litre "=1000cm^3]`
= 34.65 litres
Therefore, such vessel can hold 34.65 litres of water.
उत्तर २
`Given circumference = 2πr
`132 = 2 xx 22/7 xxr`
∴ r = 3 x 7 = 21 cm
∴ Radius = 21 cm
2) Volume of cylinder = πr2h
`= 22/7 xx 21xx21xx25`
`= 34.65 litres`
APPEARS IN
संबंधित प्रश्न
A patient in a hospital is given soup daily in a cylindrical bowl of diameter 7 cm. If the bowl is filled with soup to a height of 4 cm, how much soup the hospital has to prepare daily to serve 250 patients?
`["Assume "pi=22/7]`
Given a cylindrical tank, in which situation will you find surface area and in which situation volume.
- To find how much it can hold
- Number of cement bags required to plaster it
- To find the number of smaller tanks that can be filled with water from it.
A circus tent is cylindrical to a height of 4 m and conical above it. If its diameter is 105 m and its slant height is 80 m, calculate the total area of canvas required. Also, find the total cost of canvas used at Rs. 15 per metre if the width is 1.5 m.
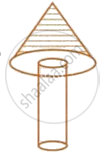
A wooden toy is in the shape of a cone mounted on a cylinder as shown alongside. If the height of the cone is 24 cm, the total height of the toy is 60 cm and the radius of the base of the cone = twice the radius of the base of the cylinder = 10 cm; find the total surface area of the toy. [Take π = 3.14]
Water flows, at 9 km per hour, through a cylindrical pipe of cross-sectional area 25 cm2. If this water is collected into a rectangular cistern of imensions 7.5 m by 5 m by 4 m; calculate therise in level in the cistern in 1 hour 15 minutes.

Calculate:
(a) the total surface area,
(b) the total volume of the solid and
(c) the density of the material if its total weight is 1.7 kg..
A metal container in the form of a cylinder is surmounted by a hemisphere of the same radius. The internal height of the cylinder is 7 m and the internal radius is 3.5 m.
Calculate:
- the total area of the internal surface, excluding the base;
- the internal volume of the container in m3.
The inner radius of a pipe is 2.1 cm. How much water can 12 m of this pipe hold?
What length of solid cylinder 2 cm in diameter must be taken to recast into a hollow cylinder of external diameter 20 cm, 0.25 cm thick and 15 cm long?
What length of solid cylinder 2 cm in diameter must be taken to recast into a hollow cylinder of external diameter 20 cm, 0.25 cm thickand 15 cm long?
Find the volume of the largest cylinder formed when a rectangular piece of paper 44 cm by 33 cm is rolled along it : longer side.
A cylindrical vessel of height 24 cm and diameter 40 cm is full of water. Find the exact number of small cylindrical bottles, each of height 10 cm and diameter 8 cm, which can be filled with this water.
The radius and height of a cylinder are in the ratio of 5 : 7 and its volume is 550 cm. Find its radius. (Take π = 22/7)
A cylindrical glass with diameter 20 cm has water to a height of 9 cm. A small cylindrical metal of radius 5 cm and height 4 cm is immersed it completely. Calculate the raise of the water in the glass?
A cone, a hemisphere and a cylinder stand on equal bases and have the same height. The ratio of their volumes is 1 : 2 : 3.
A sphere and a right circular cylinder of the same radius have equal volumes. By what percentage does the diameter of the cylinder exceed its height?
The volume of a cylinder whose radius r is equal to its height is ______.
The ratio of radii of two cylinders is 1 : 2 and heights are in the ratio 2 : 3. The ratio of their volumes is ______.
Volume of a cylinder with radius h and height r is ______.
A housing society consisting of 5,500 people needs 100 L of water per person per day. The cylindrical supply tank is 7 m high and has a diameter 10 m. For how many days will the water in the tank last for the society?
Volume of a cylinder of height 3 cm is a 48π. Radius of the cylinder is ______.
