Advertisements
Advertisements
प्रश्न
The circumferences of circular faces of a frustum are 132 cm and 88 cm and its height is 24 cm. To find the curved surface area of the frustum complete the following activity.( \[\pi = \frac{22}{7}\])
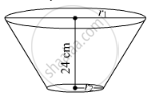
उत्तर
(Circumference)1 = 2πr1 = 132
∴ r1 = `132/(2π) = (132 xx 7)/(2 xx 22)` = 21 cm
(Circumference)1 = 2πr2 = 88
r2 = `88/(2π) = (88 xx 7)/(2 xx 22)`
= 14 cm
Slant height of frustum, `l = sqrt(h^2 + (r_1 - r_2)^2`
= `sqrt((24)^2 + (21 - 14)^2`
= `sqrt((24)^2 + (7)^2`
= `sqrt(576 + 49)` = `sqrt(625)`
= `sqrt(25 xx 25)` = 25 cm
Curved surface area of the frustum = `π(r_1 + r_2)l`
= `22/7 (21 + 14) xx 25`
= `22/7 xx 35 xx 25`
= 2750 cm2
संबंधित प्रश्न
A container, opened from the top and made up of a metal sheet, is in the form of a frustum of a cone of height 16 cm with radii of its lower and upper ends as 8 cm and 20 cm respectively. Find the cost of the milk which can completely fill the container, at the rate of Rs.20 per litre. Also find the cost of metal sheet used to make the container, if it costs Rs.8 per 100 cm2. [Take π = 3.14]
A metallic solid sphere of radius 10.5 cm is melted and recasted into smaller solid cones, each of radius 3.5 cm and height 3 cm. How many cones will be made?
Milk in a container, which is in the form of a frustum of a cone of height 30 cm and the radii of whose lower and upper circular ends are 20 cm and 40 cm respectively, is to be distributed in a camp for flood victims. If this milk is available at the rate of Rs 35 per litre and 880 litres of milk is needed daily for a camp, find how many such containers of milk are needed for a camp and what cost will it put on the donor agency for this. What value is indicated through this by the donor agency ?
The radii of ends of a frustum are 14 cm and 6 cm respectively and its height is 6 cm. Find its total surface area.
A rectangular vessel of dimensions 20 cm × 16 cm × 11 cm is full of water. This water is poured into a conical vessel. The top of the conical vessel has its radius 10 cm. If the conical vessel is filled completely, determine its height.
If a cone of radius 10 cm is divided into two parts by drawing a plane through the mid-point of its axis, parallel to its base. Compare the volumes of the two parts.
A cone, a hemisphere and a cylinder stand on equal bases and have the same height. What is the ratio of their volumes?
If r1 and r2 denote the radii of the circular bases of the frustum of a cone such that r1 > r2, then write the ratio of the height of the cone of which the frustum is a part to the height fo the frustum.
A spherical cannon ball, 28 cm in diameter, is melted and recast into a right circular conical mould with base diameter of 35 cm. Find the height of the cone.
A drinking glass is in the shape of a frustum of a cone of height 14 cm. The diameters of its two circular ends are 16 cm and 12 cm. Find the capacity of the glass.
A metallic bucket, open at the top, of height 24 cm is in the form of the frustum of a cone, the radii of whose lower and upper circular ends are 7 cm and 14 cm, respectively. Find
- the volume of water which can completely fill the bucket;
- the area of the metal sheet used to make the bucket.
A container, open at the top and made up of metal sheet, is in the form of a frustum of a cone of height 16 cm with diameters of its lower and upper ends as 16 cm and 40 cm, respectively. Find the cost of metal sheet used to make the container, if it costs ₹10 per 100 cm2
A bucket is in the form of a frustum of a cone. Its depth is 15 cm and the diameters of the top and the bottom are 56 cm and 42 cm, respectively. Find how many litres of water can the bucket hold.
A bucket is in the form of a frustum of a cone and it can hold 28.49 litres of water. If the radii of its circular ends are 28 cm and 21 cm, then find the height of the bucket.
The radii of the circular ends of a solid frustum of a cone are 33 cm and 27 cm and its slant height is 10 cm. Find its total surface area. [Use π = 3.14.]
A bucket of height 24 cm is in the form of frustum of a cone whose circular ends are of diameter 28 cm and 42 cm. Find the cost of milk at the rate of ₹30 per litre, which the bucket can hold.
A milk container is made of metal sheet in the shape of frustum of a cone whose volume is `"10459" 3/7 "cm"`. The radii of its lower and upper circular ends are 8 cm and 20 cm, respectively. Find the cost of metal sheet used in making the container at the rate of ₹1.40 per cm2.
A metallic spherical shell of internal and external diameters 4 cm and 8 cm, respectively, is melted and recast in the form of a cone of base diameter 8 cm. The height of the cone is ______.
The base radii of two circular cones of the same height are in the ratio 3 : 5. The ratio of their volumes are ______.
