Advertisements
Advertisements
प्रश्न
- The earth-moon distance is about 60 earth radius. What will be the diameter of the earth (approximately in degrees) as seen from the moon?
- Moon is seen to be of (½)°diameter from the earth. What must be the relative size compared to the earth?
- From parallax measurement, the sun is found to be at a distance of about 400 times the earth-moon distance. Estimate the ratio of sun-earth diameters.
Advertisements
उत्तर
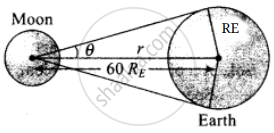
a. As the distance between moon and earth is greater than the radius of the earth, then radius of the earth can be treated as an arc.
According to the problem,
R, = length of arc
Distance between moon and earth = 60 RE
So, the angle subtended at distance r due to an arc of length `l` is
`θ_E = l/r = (2R_E)/(60 R_E) = 1/30` rad
= `1/30 xx 180^circ/pi` degree
= `6^circ/3.14` degree
= 1.9° ≈ 2°
Hence, the angle is subtended by the diameter of the earth 2θ = 2°.
b. According to the problem, the moon is seen as `(1/2)^circ` diameter from the earth and the earth is seen as 2° diameter from the moon.
As θ is proportional to diameter,
Hence, `"Diameter of earth"/"Diameter of moon" = 2/((1/2))` = 4
c. From parallax measurement given that the sun is at a distance of about 400 times the earth-moon distance, hence, `r_("sun")/r_("moon")` = 400
Sun and moon both appear to be of the same angular diameter as seen from the earth. (Suppose, here r stands for distance and D for diameter)
∴ `D_(sun)/r_(sun) = D_("moon")/r_("moon")`
⇒ `D_(sun)/D_("moon")` = 400
But `D_(earth)/D_("moon")` = 4
⇒ `D_(sun)/D_(earth)` = 100
APPEARS IN
संबंधित प्रश्न
The principle of ‘parallax’ in section 2.3.1 is used in the determination of distances of very distant stars. The baseline AB is the line joining the Earth’s two locations six months apart in its orbit around the Sun. That is, the baseline is about the diameter of the Earth’s orbit ≈ 3 × 1011m. However, even the nearest stars are so distant that with such a long baseline, they show parallax only of the order of 1” (second) of arc or so. A parsec is a convenient unit of length on the astronomical scale. It is the distance of an object that will show a parallax of 1” (second) of arc from opposite ends of a baseline equal to the distance from the Earth to the Sun. How much is a parsec in terms of meters?
Just as precise measurements are necessary in science, it is equally important to be able to make rough estimates of quantities using rudimentary ideas and common observations. Think of ways by which you can estimate the following (where an estimate is difficult to obtain, try to get an upper bound on the quantity):
the number of strands of hair on your head
Just as precise measurements are necessary in science, it is equally important to be able to make rough estimates of quantities using rudimentary ideas and common observations. Think of ways by which you can estimate the following (where an estimate is difficult to obtain, try to get an upper bound on the quantity):-
the number of air molecules in your classroom.
Answer the following question.
Star A is farther than star B. Which star will have a large parallax angle?
While measuring the length of an object using a ruler, the position of your eye should be
Metre is the unit of ______.
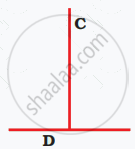
Which line is longer? C or D? Measure each line. How good is your guess?
Measure the yellow rectangle. It is ________ cm long.
How many stamps can be placed along its length?
There are two beautiful lakes near a village. People come for boating and picnics in both the lakes. The village Panchayat is worried that with the noise of the boats the birds will stop coming. The Panchayat wants motorboats in only one lake. The other lake will be saved for the birds to make their nests.
- How many kilometers long is the actual boundary of lake B?
Length is a fundamental quantity.
The distance between one end and the other end is called ______.
Length is ______.
SI unit of electric current is ______.
To measure the distance of a planet from the earth, ______ method is used.
In an experiment to estimate the size of a molecule of oleic acid 1 mL of oleic acid is dissolved in 19 mL of alcohol. Then 1 mL of this solution is diluted to 20 mL by adding alcohol. Now 1 drop of this diluted solution is placed on water in a shallow trough. The solution spreads over the surface of water forming one molecule thick layer. Now, lycopodium powder is sprinkled evenly over the film and its diameter is measured. Knowing the volume of the drop and area of the film we can calculate the thickness of the film which will give us the size of oleic acid molecule.
Read the passage carefully and answer the following questions:
- Why do we dissolve oleic acid in alcohol?
- What is the role of lycopodium powder?
- What would be the volume of oleic acid in each mL of solution prepared?
- How will you calculate the volume of n drops of this solution of oleic acid?
- What will be the volume of oleic acid in one drop of this solution?
How many astronomical units (A.U.) make 1 parsec?
What is an Astronomical Unit (AU)?
Why is the speed of light used to define the metre?
