Advertisements
Advertisements
प्रश्न
The given figure shows a parallelogram ABCD. E is a point in AD and CE produced meets BA produced at point F. If AE = 4 cm, AF = 8 cm and AB = 12 cm, find the perimeter of the parallelogram ABCD.

उत्तर
AF = 8 cm and AB = 12cm
So, FB = 20 cm
In ΔDEC and ΔEAF
∠DEC = ∠EAF ...(Vertically opposite angles)
∠EDC = ∠EAF ...(Alternate angles)
So, ΔDEC ∼ ΔAEF ...(AA criterion for similarity)
`=> (DE)/(AE) = (EC)/(EF) = (DC)/(AF)`
`=> (DE)/(AE) = (DC)/(AF)`
`=> (DE)/(AE) = (AB)/(AF)`
`=> (DE)/4 = 12/8`
`=>` DE = 6 cm
So, AD = AE + ED
= 4 + 6
= 10 cm
Perimeter of the parallelogram ABCD
= AB + BC + CD + AD
= 12 + 10 + 12 + 10
= 44 cm
Notes
Δ
APPEARS IN
संबंधित प्रश्न
See the given figure. DE || BC. Find AD.

In the given figure, QR is parallel to AB and DR is parallel to AB and DR is parallel to QB.

Prove that: PQ2 = PD × PA.
In the given figure, BC is parallel to DE. Area of triangle ABC = 25 cm2, Area of trapezium BCED = 24 cm2 and DE = 14 cm. Calculate the length of BC. Also, find the area of triangle BCD.

In each of the given pairs of triangles, find which pair of triangles are similar. State the similarity criterion and write the similarity relation in symbolic form:

In ΔABC, point D divides AB in the ratio 5:7, Find: `"AE"/"AC"`
If ΔPQR, AB is drawn parallel to QR. If PQ = 9cm, PR = 6cm and PB = 4.cm, find the length of AP.
A model of a ship is made to a scale of 1:500. Find: The area other deck o the ship, if the area of the deck of its model is m2
The perimeters of two similar triangles ∆ABC and ∆PQR are 36 cm and 24 cm respectively. If PQ = 10 cm, then the length of AB is
From the figure, prove that ∆SUN ~ ∆RAY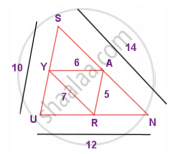
It is given that ΔABC ~ ΔPQR, with `(BC)/(QR) = 1/3`. Then, `(ar(PRQ))/(ar(BCA))` is equal to ______.
