Advertisements
Advertisements
प्रश्न
The number of particles present in Face Centred Cubic Unit cell is/are ______.
पर्याय
1
2
3
4
उत्तर
The number of particles present in Face Centred Cubic Unit cell is 4.
APPEARS IN
संबंधित प्रश्न
Answer the following in brief.
Calculate the number of atoms in fcc unit cell.
Obtain the relationship between the density of a substance and the edge length of the unit cell.
Write the relationship between radius of atom and edge length of fcc unit cell.
Give the percentage of empty space in bcc lattice.
If the total volume of a simple cubic unit cell is 6.817 × 10-23 cm3, what is the volume occupied by particles in the unit cell?
Calculate the number of unit cells in 0.3 g of a species having density of 8.5 g/cm3 and unit cell edge length 3.25 × 10-8 cm.
Derive the relationship between density of substance, its molar mass, and the unit cell edge length. Explain how you will calculate the number of particles, and a number of unit cells in x g of metal.
An element crystallizes in fcc type of unit cell. The volume of one unit cell is 24.99 × 10-24 cm3 and density of the element 7.2 g cm-3, Calculate the number of unit cells in 36 g of pure sample of element?
What is the percentage of unoccupied space in fcc unit cell?
How many total constituent particles are present in simple cubic unit cell?
The number of atoms in 500 g of a fcc crystal of a metal with density d = 10 g/cm3 and cell edge 100 pm, is equal to ____________.
A metal crystallises in bcc unit cell with edge length 'a'. What will be the volume of one atom?
An element with density 2.8 g cm−3 forms fcc unit cell having edge length 4 × 10−8 cm. Calculate molar mass of the element.
Copper and silver have ____________ crystal structure.
Consider the following unit cell.
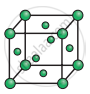
The number of particles (spheres) per unit cell is:
What is the density of iron crystal which crystallizes in body-centred cubic structure with edge length 287 pm? (At. mass of Fe = 56 amu)
The number of atoms in 100 g of an fcc crystal with density 10 g cm-3 and unit cell edge length 200 pm is equal to ______.
The coordination number of atoms in body-centred cubic structure (bcc) is ______.
Gold crystallises into face-centred cubic cells. The edge length of a unit cell is 4.08 × 10–8 cm. Calculate the density of gold. [Molar mass of gold = 197 g mol–1]
An element has a bee structure with unit cell edge length of 288 pm. How many unit cells and number of atoms are present in 200 g of the element?
A metal has an fcc lattice. The edge length of the unit cell is 404 pm. The density of the metal is 2.72 g cm−3. The molar mass of the metal is ______.
(NA Avogadro's constant = 6.02 × 1023 mol−1)
An element with molar mass 2.7 × 10-2 kg/mol. Forms a cubic units cell with edge length of 405 pm. If the density is 2.7 × 103 kg/m3. Find the nature of a cubic unit cell.
What is base centred (or end-centred) unit cell?
The correct sequence of the atomic layers in cubic close packing is ______.
An element crystallises in fee structure. If molar mass of element is 72.7 g mol -1, the mass of its one unit cell will be ______.
