Advertisements
Advertisements
प्रश्न
The numerical value of the area of a circle is greater than the numerical value of its circumference. Is this statement true? Why?
उत्तर
Let r be the radius of the circle.
Area of the circle = πr2
Circumference of the circle = 2πr
Both are equal only when r = 2 and numerical value of circumference is greater than numerical value of area of circle when 0 < r < 2 and if r > 2, then numerical value of area of circle is greater than the numerical value of the circumference of the circle.
APPEARS IN
संबंधित प्रश्न
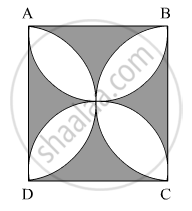
In Fig. 4, ABCD is a square of side 14 cm. Semi-circles are drawn with each side of square as diameter. Find the area of the shaded region.
(use `pi=22/7`)
In Figure, find the area of the shaded region [Use π = 3.14]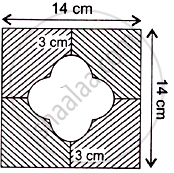
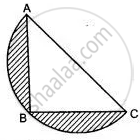
ABC is an isosceles right-angled triangle with ∠ABC = 90°. A semi-circle is drawn with AC as the diameter. If AB = BC = 7 cm, find the area of the shaded region. [Take π = 22/7]
Find the angle subtended at the centre of circle of radius 5cm by an arc of length `((5pi)/3)` cm
The hypotenuse of a right-angled triangle is 65 cm and its base is 60 cm. Find the length of perpendicular and the area of the triangle.
The area of the circle is given below; find the diameter.
176 sq cm
Find the volume and the surface area of the spheres in the following :
Radius= 2.1 cm
Each side of a square is 44 cm. Find its perimeter. If this perimeter is equal to the circumference of a circle, find the radius of the circle.
Sudhanshu divides a circular disc of radius 7 cm in two equal parts. What is the perimeter of each semicircular shape disc? (Use π = `22/7`)
A ground is in the form of a circle whose diameter is 350 m. An athlete makes 4 revolutions. Find the distance covered by the athlete
