Question

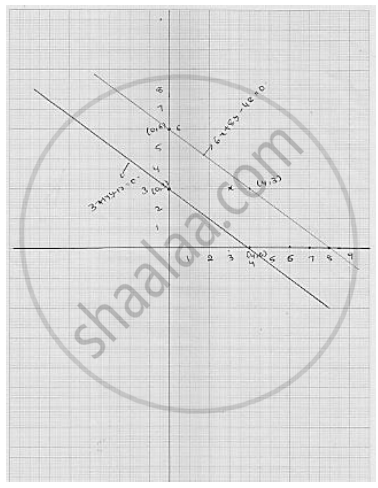
The path of a train A is given by the equation 3x + 4y − 12 = 0 and the path of another train
B is given by the equation 6x + 8y − 48 = 0. Represent this situation graphically.

Answer 1

We have 

        3x + 4y - 12 = 0 

 ⇒    3x = 12  - 4y 

 ⇒     `3x  = ( 12 - 4y ) /3 `

Putting  y  = 0  We get ` x (12 - 4 xx 0 ) /3= 4 `

Putting  y = 3 , we get  ` x  = (12 - 4 xx 0 ) / 3 = 0 ` 

Thus, we have the following table for the points on the line  3x + 4y - 12 =  0 

x	4	0
y	0	3

We have 

 6x + 8y - 48 = 0 

6x + 8y = 48 

6x = 48 - 8y 

`x = 48 - (8y )/ 6 `

Putting  y = 6 , we get  ` x = ( 48 - 8 xx 6) / 6 = 0`

 Putting y = 4 we get ` x = (48 - 8 xx 3) / 6= 4`

Thus, we have the following table for the points on the line 6x + 8y - 48 = 0

x	0	4
y	6	3