Advertisements
Advertisements
प्रश्न
The radius of planet A is half the radius of planet B. If the mass of A is MA, what must be the mass of B so that the value of g on B is half that of its value on A?
उत्तर १
The acceleration due to gravity of a planet is given as
\[\text{g} = \frac{\text{GM}}{\text{r}^2}\]
For planet A:
For planet B:
\[\text{g}_{B} = \frac{\text{GM}_\text{B}}{\text{r}_\text{B}^2}\]
\[\text{g}_\text{B} = \frac{1}{2} \text{g}_\text{A}\] ...(Given) or,
\[\frac{\text{GM}_\text{B}}{\text{r}_\text{B}^2} = \frac{\text{G M}_\text{A}}{2 \text{r}_\text{A}^2}\]
\[\Rightarrow \text{M}_\text{B} = \frac{\text{M}_\text{A} \text{r}_\text{B}^2}{2 \text{r}_\text{A}^2}\]
\[\Rightarrow \text{M}_\text{B} = \frac{\text{M}_\text{A} \text{r}_\text{B}^2}{2(\frac{1}{2} \text{r}_\text{B})^2} = 2 \text{M}_\text{A}\]
उत्तर २
radius of planet ‘A’ = RA, radius of planet ‘B’ = RB
Mass of planet ‘A’ = MA, mass of planet ‘B’ = MB = ?
From given...
`"R"_"A" = ("R"_"B")/2; "g"_"B" = 1/2 "g"_"A"`
`"g" = ("GM")/("R"^2);`
`∴ "g"_"A" = ("GM"_"A")/("R"_"A"^2)`;
`∴ "g"_"B" = ("GM"_"B")/("R"_"B"^2)`
`("GM"_"B")/("R"_"B"^2)`
`("M"_"B")/("R"_"B"^2) = 1/2(("GM"_"A")/(("RB"/2)^2))`
`("M"_"B")/("R"_"B"^2) = 1/2 (4("GM"_"A")/(("R"_"B")^2))`
`"M"_"B" = 2 "M"_"A"`
APPEARS IN
संबंधित प्रश्न
Fill in the following blank with suitable word :
The value of g on the earth is about………………. of that on the moon.
If the value of g suddenly becomes twice its value, it will become two times more difficult to pull a heavy object along the floor. Why?
An object takes 5 s to reach the ground from a height of 5 m on a planet. What is the value of g on the planet?
Why does the velocity of a stone thrown vertically upwards decreases?
The CGS unit of G is dyne.cm2/g2.
Write scientific reason.
The value of acceleration g is greater at the pole than at the equator.
Write scientific reason.
The value of gravitational acceleration (g) decreases as we go above the Earth's surface.
Write scientific reason.
The weight of an object varies on different planets.
The mass of a body on the surface of the earth is 10 kg. The mass of the same body on the surface on the moon is `"g"_"m" = 1/6 "g"_"e"`, where gm, ge acceleration due to gravity on the surface of the moon and the earth respectively.
The acceleration due to gravity on moon is `(1/6)^"th"` times the acceleration due to gravity on earth. If the ratio of the density of earth 'ρe' to the density of moon 'ρm' is `5/3`, then the radius of moon 'Rm' in terms of the radius of earth 'Re' is ______.
When the value of acceleration due to gravity 'g' becomes `(g/3)` above the earth's surface at height 'h' then relation between 'h' and 'R' is ______.
R =radius of the earth
How does the force of attraction between the two bodies depend upon their masses and the distance between them? A student thought that two bricks tied together would fall faster than a single one under the action of gravity. Do you agree with his hypothesis or not? Comment.
A central particle M is surrounded by a square array of other particles, separated by either distanced or distance d/2 along the perimeter o the square. The magnitude of the gravitational force on the central particle due to the other particles is ______.

A mass attached to one end of a string crosses top-most point on a vertical circle with critical speed. Its centripetal acceleration when string becomes horizontal will be ______. (g = gravitational acceleration)
A lift of mass 'm' is connected to a rope which is moving upward with maximum acceleration 'a'. For maximum safe stress, the elastic limit of the rope is 'T'. The minimum diameter of the rope is ______.
(g = gravitational acceleration)
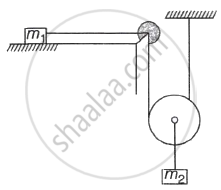
If the surface is smooth, the acceleration of the block m2 will be ______.
If the change in the value of g at the height h above the surface of the earth is the same as at a depth x below it, then ______.
(both x and h being much smaller than the radius of the earth)
A uniform ring of mass M and radius r is placed directly above a uniform sphere of mass 8M and of same radius R. The centre of the ring is at a distance of d = `sqrt3`R from the centre of the sphere. The gravitational attraction between the sphere and the ring is ______.
