Advertisements
Advertisements
प्रश्न
There is a ladder of length 32 m which rests on a pole. If the height of pole is 18 m, determine the distance between the foot of ladder and the pole.
उत्तर
In the figure, AC is the ladder and AB is the pole.
So, the distance between the foot of ladder and pole is BC.
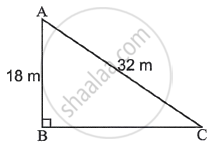
According to the Pythagoras theorem,
AB2 + BC2 = AC2 ......(i)
Substituting the values for AB and AC in equation (i),
182 + BC2 = 322
⇒ 324 + BC2 = 1024
⇒ BC2 = 1024 – 324
⇒ BC2 = 700
⇒ BC = `sqrt(700)` = `10sqrt(7)`
As a result, the distance between the ladder's foot and the pole is `10sqrt(7)`m.
APPEARS IN
संबंधित प्रश्न
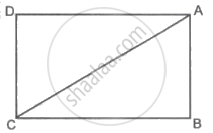
In the above figure `square`ABCD is a rectangle. If AB = 5, AC = 13, then complete the following activity to find BC.
Activity: ΔABC is a `square` triangle.
∴ By Pythagoras theorem
AB2 + BC2 = AC2
∴ 25 + BC2 = `square`
∴ BC2 = `square`
∴ BC = `square`
Construct two concentric circles with centre O with radii 3 cm and 5 cm. Construct a tangent to a smaller circle from any point A on the larger circle. Measure and write the length of the tangent segment. Calculate the length of the tangent segment using Pythagoras' theorem.
In the right-angled triangle ABC, Hypotenuse AC = 10 and side AB = 5, then what is the measure of ∠A?
If tan θ = `12/5`, then 5 sin θ – 12 cos θ = ?
From the information in the figure, complete the following activity to find the length of the hypotenuse AC.

AB = BC = `square`
∴ ∠BAC = `square`
Side opposite angle 45° = `square/square` × Hypotenuse
∴ `5sqrt(2) = 1/square` × AC
∴ AC = `5sqrt(2) xx square = square`
AB, BC and AC are three sides of a right-angled triangle having lengths 6 cm, 8 cm and 10 cm, respectively. To verify the Pythagoras theorem for this triangle, fill in the boxes:
ΔABC is a right-angled triangle and ∠ABC = 90°.
So, by the Pythagoras theorem,
`square` + `square` = `square`
Substituting 6 cm for AB and 8 cm for BC in L.H.S.
`square` + `square` = `square` + `square`
= `square` + `square`
= `square`
Substituting 10 cm for AC in R.H.S.
`square` = `square`
= `square`
Since, L.H.S. = R.H.S.
Hence, the Pythagoras theorem is verified.
A person starts his trip from home. He moves 24 km in south direction and then starts moving towards east. He travels 7 km in that direction and finally reaches his destination. How far is the destination from his home?
Find the side of a square whose diagonal is `10sqrt2` cm.
