Advertisements
Advertisements
प्रश्न
Two charges of magnitudes +4Q and − Q are located at points (a, 0) and (− 3a, 0) respectively. What is the electric flux due to these charges through a sphere of radius ‘2a’ with its centre at the origin?
उत्तर
Gauss’ theorem states that the electric flux through a closed surface enclosing a charge is equal to `1/epsi_0`times the magnitude of the charge enclosed.
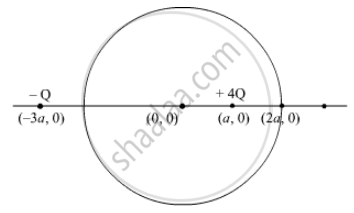
The sphere enclose charge = +4Q.
Thus,`phi = (4Q)/epsi_0`
APPEARS IN
संबंधित प्रश्न
Find out the outward flux to a point charge +q placed at the centre of a cube of side ‘a’. Why is it found to be independent of the size and shape of the surface enclosing it? Explain.
What is the electric flux through a cube of side 1 cm which encloses an electric dipole?
Define Electric Flux. Write its SI unit.
Given a uniform electric filed \[\vec{E} = 4 \times {10}^3 \ \hat{i} N/C\]. Find the flux of this field through a square of 5 cm on a side whose plane is parallel to the Y-Z plane. What would be the flux through the same square if the plane makes a 30° angle with the x-axis?
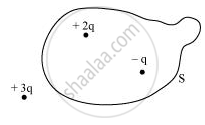
Figure shows three point charges +2q, −q and + 3q. Two charges + 2q and −q are enclosed within a surface ‘S’. What is the electric flux due to this configuration through the surface ‘S’?
Choose the correct answer from given options
The electric flux through a closed Gaussian surface depends upon
A cylinder of radius R and length L is placed in a uniform electric field E parallel to the cylinder axis. The total flux for the surface of the cylinder is given by ______.
A charge Qµc is placed at the centre of a cube the flux coming from any surface will be.
An electric charge q is placed at the center of a cube of side ℓ. The electric flux on one of its faces will be ______.
