Advertisements
Advertisements
प्रश्न
Two opposite angles of a parallelogram are (3x − 2)° and (50 − x)°. Find the measure of each angle of the parallelogram.
उत्तर
\[\text{ Oppostie angles of a parallelogram are congurent } . \]
\[ \therefore \left( 3x - 2 \right)° = \left( 50 - x \right)°\]
\[3x°- 2° = 50°- x°\]
\[3x°+ x°= 50° + 2°\]
\[4x°= 52°\]
\[x° = 13°\]
\[\text{ Putting the value of x in one angle }: \]
\[3x° - 2°= 39°- 2°\]
\[ = 37°\]
\[\text{ Opposite angles are congurent }: \]
\[ \therefore 50 - x°\]
\[ = 37°\]
\[\text{ Let the remaining two angles be y and z } . \]
\[\text{ Angles y and z are congurent because they are also opposite angles } . \]
\[ \therefore y = z\]
\[\text{ The sum of adjacent angles of a paralle\logram is equal to } 180° . \]
\[ \therefore 37°+ y = 180°\]
\[y = 180°- 37°\]
\[y = 143°\]
\[\text{ So, the anlges measure are }: \]
\[37°, 37°, 143° \text{ and } 143°\]
APPEARS IN
संबंधित प्रश्न
All the angles of a quadrilateral are equal to each other. Find the measure of each. Is the quadrilateral a parallelogram? What special type of parallelogram is it?
Find the angles marked with a question mark shown in Fig. 17.27

In the following Figure ABCD is a arallelogram, CE bisects ∠C and AF bisects ∠A. In each of the following, if the statement is true, give a reason for the same:
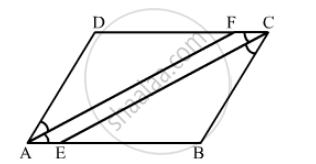
(i) ∠A = ∠C
(ii) \[\angle FAB = \frac{1}{2}\angle A\]
(iii) \[\angle DCE = \frac{1}{2}\angle C\]
(iv) \[\angle CEB = \angle FAB\]
(v) CE || AF
Which of the following statement is true for a rhombus?
Its diagonals bisect each other at right angles.
Which of the following statement is true for a rhombus?
It is a square.
Fill in the blank, in each of the following, so as to make the statement true:
If the diagonals of a parallelogram bisect each other at right angles, then it is a ......
Measure of one angle of a rhombus is 50°, find the measures of remaining three angles.
If the diagonal of a rhombus are equal, then the rhombus is a
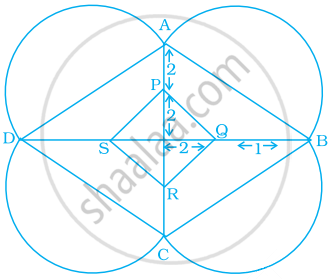
A Rangoli has been drawn on a flor of a house. ABCD and PQRS both are in the shape of a rhombus. Find the radius of semicircle drawn on each side of rhombus ABCD.
Construct a rhombus whose side is 5 cm and one angle is of 60°.
