Advertisements
Advertisements
प्रश्न
Two resistors R1= 60 Ω and R2 = 90Ω are connected in parallel. If electric power consumed by the resistor R1 is15 W, calculate the power consumed by the resistor R2.
उत्तर
Power of R1=`(V^2)/(R_1)`
⇒ `15 = (V^2)/60`
⇒ `V^2 = 60 xx 15 = 900`
∴ V = 30 V
Hence, R2 being parallel with R1, has same p.d.
∴ Power of `R_2 =(30xx30)/90 `= 10W.
APPEARS IN
संबंधित प्रश्न
Three resistors 1 Ω, 2 Ω, and 3 Ω are combined in series. What is the total resistance of the combination?
Three resistors 2 Ω, 4 Ω and 5 Ω are combined in parallel. What is the total resistance of the combination?
Two heating elements of resistances R1 and R2 when operated at a constant supply of voltage, V, consume powers P1 and P2 respectively. Deduce the expressions for the power of their combination whey they are, in turn, connected in (i) series and (ii) parallel across the same voltage supply.
Suppose you have three resistors of 20 Ω, 50 Ω and 100 Ω. What minimum and maximum resistance can you obtain from these resistors?
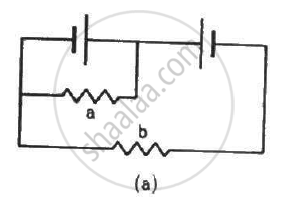
Each of the resistors shown in the figure has a resistance of 10 Ω and each of the batteries has an emf of 10 V. Find the currents flowing through the resistors a and bin the two circuits.

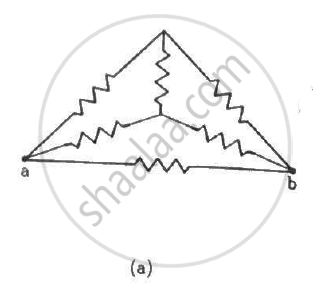
Find the equivalent resistance of the circuits shown in the figure between the points a and b. Each resistor has resistance r.

To get maximum current in a resistance of 3 ohms, one can use n rows of m cells (connected in series) connected in parallel. If the total number of cells is 24 and the internal resistance of a cell is 0.5 ohms then ______.
Two electric lamps of each 40 watt are connected in series. The power consumed by the combination will be
The equivalent resistance of resistors connected in series is always ______
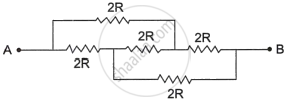
- Assertion (A): The equivalent resistance between points A and B in the given networks is 2R.
- Reason (R): All the resistors are connected in parallel.