Advertisements
Advertisements
प्रश्न
Use graph paper for this question. Take 2 cm = 2 units on x-axis and 2 cm = 1 unit on y-axis.
Solve graphically the following equation:
3x + 5y = 12; 3x - 5y + 18 = 0 (Plot only three points per line)
उत्तर
3x + 5y = 12
⇒ 3x = 12 - 5y
x = `(12 - 5y)/(3)`
The table for 3x + 5y = 12 is
| X | 4 | - 1 | - 6 |
| Y | 0 | 3 | 6 |
Also we have
3x - 5y + 18 = 0
⇒ 3x = 5y - 18
⇒ x = `(5y - 18)/(3)`
The table for 3x - 5y + 18 = 0 is
| X | - 6 | 4 | - 1 |
| Y | 0 | 6 | 3 |
Plotting the above points we get the following required graph:
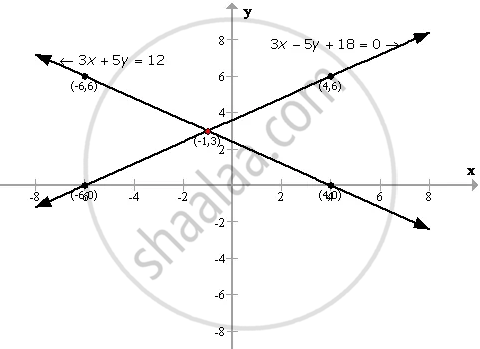
From the above graph, it is clear that the two lines 3x + 5y = 12 and 3x - 5y + 18 = 0 intersect at the point (-1, 3)
APPEARS IN
संबंधित प्रश्न
In countries like USA and Canada, temperature is measured in Fahrenheit, whereas in countries like India, it is measured in Celsius. Here is a linear equation that converts Fahrenheit to Celsius:-
`F=(9/5)C+32`
(i) Draw the graph of the linear equation above using Celsius for x-axis and Fahrenheit for y-axis.
(ii) If the temperature is 30°C, what is the temperature in Fahrenheit?
(iii) If the temperature is 95°F, what is the temperature in Celsius?
(iv) If the temperature is 0°C, what is the temperature in Fahrenheit and if the temperature is 0°F, what is the temperature in Celsius?
(v) Is there a temperature which is numerically the same in both Fahrenheit and Celsius? If yes, find it.
Draw the graph of the following linear equation in two variable : y = 2x
Draw the graph of the equation given below. Also, find the coordinates of the point
where the graph cuts the coordinate axes : 6x − 3y = 12
A straight line passes through the points (2, 4) and (5, - 2). Taking 1 cm = 1 unit; mark these points on a graph paper and draw the straight line through these points. If points (m, - 4) and (3, n) lie on the line drawn; find the values of m and n.
Draw the graphs of the following linear equations:
y + 5 = 0
Draw the graph for the following
y = `(3/2)x + 3`
Draw the graph for the following
3x + 2y = 14
A pair of linear equations has no solution then the graphical representation is
Draw the graph of the linear equation whose solutions are represented by the points having the sum of the coordinates as 10 units.
The force exerted to pull a cart is directly proportional to the acceleration produced in the body. Express the statement as a linear equation of two variables and draw the graph of the same by taking the constant mass equal to 6 kg. Read from the graph, the force required when the acceleration produced is (i) 5 m/sec2, (ii) 6 m/sec2.
