Advertisements
Advertisements
प्रश्न
Draw the graph of the linear equation whose solutions are represented by the points having the sum of the coordinates as 10 units.
उत्तर
As per question, the sum of the coordinates is 10 units.
Let x and y be two coordinates, then we get x + y = 10.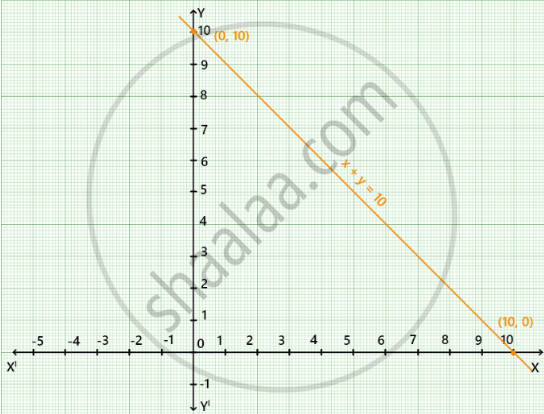
For x = 5, y = 5, therefore, (5, 5) lies on the graph of x + y = 10.
For x = 3, y = 7, therefore, (3, 7) lies on the graph of x + y = 10.
Now, plotting the points (5, 5) and (3, 7) on the graph paper and joining them by a line, we get graph of the linear equation x + y = 10.
APPEARS IN
संबंधित प्रश्न
Draw the graph of the following linear equation in two variables:
x + y = 4
Draw the graph of the equation given below. Also, find the coordinates of the point
where the graph cuts the coordinate axes : −x + 4y = 8
Draw the graph for the equation, given below :
3x + 2y = 6
A straight line passes through the points (2, 4) and (5, - 2). Taking 1 cm = 1 unit; mark these points on a graph paper and draw the straight line through these points. If points (m, - 4) and (3, n) lie on the line drawn; find the values of m and n.
Use graph paper for this question. Take 2 cm = 2 units on x-axis and 2 cm = 1 unit on y-axis.
Solve graphically the following equation:
3x + 5y = 12; 3x - 5y + 18 = 0 (Plot only three points per line)
Using a scale of 1 cm to 1 unit for both the axes, draw the graphs of the following equations: 6y = 5x + 10, y = 5x - 15.
From the graph find :
(i) the coordinates of the point where the two lines intersect;
(ii) the area of the triangle between the lines and the x-axis.
Draw the graph for the following
y = 4x – 1
Draw the graph of y = x – 4
Draw the graph of y = 2x + 5
The point (0, 3) lies on the graph of the linear equation 3x + 4y = 12.
