Advertisements
Advertisements
प्रश्न
Using Heron’s formula, find the area of a triangle whose sides are 10 cm, 24 cm, 26 cm
उत्तर
Let a = 10 cm, b = 24 cm and c = 26 cm
s = `("a" + "b" + "c")/2`
= `(10 + 24 + 26)/2`
s = `60/2`
= 30 cm
s – a = 30 – 10 = 20 cm
s – b = 30 – 24 = 6 cm
s – c = 30 – 26 = 4 cm
Area of a triangle
= `sqrt("s"("s" - "a")("s" - "b")("s" - "c"))`
= `sqrt(30 xx 20 xx 6 xx 4)`
= `sqrt(2 xx 3 xx 5 xx 2^2 xx 5 xx 2 xx 3 xx 2^2)`
= `sqrt(2^6 xx 3^2 xx 5^2)`
= 23 × 3 × 5
= 8 × 3 × 5
= 120 cm2
Area of a triangle = 120 cm2
APPEARS IN
संबंधित प्रश्न
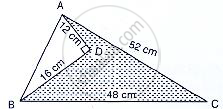
Find the area of the shaded region in the given figure.
A rhombus sheet, whose perimeter is 32 m and whose one diagonal is 10 m long, is painted on both sides at the rate of Rs 5 per m2. Find the cost of painting.
The adjacent sides of a parallelogram ABCD measure 34 cm and 20 cm, and the diagonal AC measures 42 cm. Find the area of the parallelogram.
Find the area of a triangle whose base and altitude are 5 cm and 4 cm respectively.
Sides of a triangle are cm 45 cm, 39 cm and 42 cm, find its area.
Determine whether the sets of points are collinear?
`(-1/2, 3), (-5, 6) and (-8, 8)`
If the points A(– 3, 9), B(a, b) and C(4, – 5) are collinear and if a + b = 1, then find a and b
The perimeter of an equilateral triangle is 30 cm. The area is
The area of the isosceles triangle is `5/4 sqrt(11)` cm2, if the perimeter is 11 cm and the base is 5 cm.
The perimeter of an isosceles triangle is 32 cm. The ratio of the equal side to its base is 3 : 2. Find the area of the triangle.
