Advertisements
Advertisements
प्रश्न
We have 0.5 g of hydrogen gas in a cubic chamber of size 3 cm kept at NTP. The gas in the chamber is compressed keeping the temperature constant till a final pressure of 100 atm. Is one justified in assuming the ideal gas law, in the final state?
(Hydrogen molecules can be consider as spheres of radius 1 Å).
उत्तर
Assuming hydrogen molecules as spheres of radius 1 Å.
So, r = 1 Å = radius
The volume of hydrogen molecules = `4/3 pir^3`
= `4/3 (3.14)(10^-10)^3`
= `4 xx 10^-30 m^3`
Number of moles of H2 = `"Mass"/"Molecular mass"`
= `0.5/2`
= 0.25
Molecules of H2 present = Number of moles of H2 present × 6.023 × 1023
= 0.25 × 6.023 × 1023
∴ Volume of molecules present = Molecules number × Volume of each molecule
= 0.25 × 6.023 × 1023 × 4 × 10–30
= 6.023 × 1023 × 10–30
= 6 × 10–7 m3 ......(i)
Now, if the ideal gas law is considered to be constant,
`p_iV_i = p_fV_f`
`V_f = (p_i/p_f)`
`V_i = (1/100)(3 xx 10^-2)^3`
= `(27 xx 10^-6)/10^2`
= 2.7 × 10–7 m3 ......(ii)
Hence, on compression, the volume of the gas is of the order of the molecular volume [form equation (i) and equation (ii)]. The intermolecular forces will play a role and the gas will deviate from ideal gas behaviour.
APPEARS IN
संबंधित प्रश्न
The energy of a given sample of an ideal gas depends only on its
Which of the following quantities is zero on an average for the molecules of an ideal gas in equilibrium?
Keeping the number of moles, volume and temperature the same, which of the following are the same for all ideal gases?
Consider the quantity \[\frac{MkT}{pV}\] of an ideal gas where M is the mass of the gas. It depends on the
The ratio of the molar heat capacities of an ideal gas is Cp/Cv = 7/6. Calculate the change in internal energy of 1.0 mole of the gas when its temperature is raised by 50 K (a) keeping the pressure constant (b) keeping the volume constant and (c) adiaba
An ideal gas is taken through a process in which the pressure and the volume are changed according to the equation p = kV. Show that the molar heat capacity of the gas for the process is given by `"C" ="C"_"v" +"R"/2.`
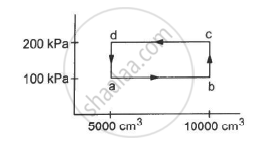
Half mole of an ideal gas (γ = 5/3) is taken through the cycle abcda, as shown in the figure. Take `"R" = 25/3"J""K"^-1 "mol"^-1 `. (a) Find the temperature of the gas in the states a, b, c and d. (b) Find the amount of heat supplied in the processes ab and bc. (c) Find the amount of heat liberated in the processes cd and da.
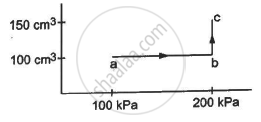
An ideal gas (γ = 1.67) is taken through the process abc shown in the figure. The temperature at point a is 300 K. Calculate (a) the temperatures at b and c (b) the work done in the process (c) the amount of heat supplied in the path ab and in the path bcand (d) the change in the internal energy of the gas in the process.
Consider a given sample of an ideal gas (Cp/Cv = γ) having initial pressure p0 and volume V0. (a) The gas is isothermally taken to a pressure p0/2 and from there, adiabatically to a pressure p0/4. Find the final volume. (b) The gas is brought back to its initial state. It is adiabatically taken to a pressure p0/2 and from there, isothermally to a pressure p0/4. Find the final volume.
Diatomic molecules like hydrogen have energies due to both translational as well as rotational motion. From the equation in kinetic theory `pV = 2/3` E, E is ______.
- the total energy per unit volume.
- only the translational part of energy because rotational energy is very small compared to the translational energy.
- only the translational part of the energy because during collisions with the wall pressure relates to change in linear momentum.
- the translational part of the energy because rotational energies of molecules can be of either sign and its average over all the molecules is zero.
