Advertisements
Advertisements
प्रश्न
What do you mean by power rating of an electrical appliance? How do you use it to calculate
(a) the resistance of the appliance and
(b) the safe limit of the current in it, while in use?
उत्तर
An electrical appliance such as electric bulb, geyser etc. is rated with power (P) and voltage (V) which is known as its power rating. For example: If an electric bulb is rated as 50W-220V, it means that when the bulb is lighted on a 220 V supply, it consumes 50 W electrical power.
(a) To calculate the resistance of the appliance, the expression is:
Resisteance , R = `"V"^2/"P"`
(b) The safe limit of current I is : I = `"P"/"V"`
APPEARS IN
संबंधित प्रश्न
How does the resistance of a pure metal change if its temperature decreases?
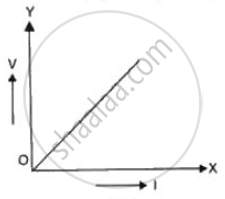
A graph is plotted taking p.d. along y-axis and electric current along x-axis. Name the physical quantity that represents the slope of this graph (Fig.).
Choose the correct option from given alternative:
Two bulbs of 100 W and 40 W are connected in series. The current through the 100 W bulb is 1A. The current through the 40W bulb will be:
Bends in a rubber pipe reduce the flow of water through it. How would the bands in a wire affect its electric resistance?
Calculate the equivalent resistance between P and Q from the following diagram:
Two lamps of resistance 30Ω and 20Ω respectively are connected in series in a 110V circuit. Calculate:
(i) the total resistance in the circuit
(ii) the current in the circuit, and
(iii) the voltage drop across each lamp.
With the help of a circuit diagram derive the formula for the resultant resistance of three resistances connected:
- in series and
- in parallel
If the current I through a resistor is increased by 100% (assume that temperature remains unchanged), the increase in power dissipated will be:
When a 4 Ω resistor is connected across the terminals of a 2 V battery, the number of coulombs passing through the resistor per second is:
Two electric bulbs have resistances in the ratio 1 : 2. If they are joined in series, the energy consumed in them is in the ratio:
