Advertisements
Advertisements
A roller of diameter 0.9 m and the length 1.8 m is used to press the ground. Find the area of the ground pressed by it in 500 revolutions.
`(pi=3.14)`
Concept: Properties of Ratios of Areas of Two Triangles
Draw the circumcircle of ΔPMT in which PM = 5.6 cm, ∠P = 60°, ∠M = 70°.
Concept: Property of an Angle Bisector of a Triangle
The ratio of corresponding sides of similar triangles is 3 : 5, then find the ratio of their areas.
Concept: Areas of Similar Triangles
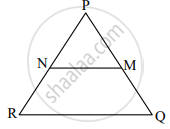
In ΔPQR, NM || RQ. If PM = 15, MQ = 10, NR = 8, then find PN.
Concept: Basic Proportionality Theorem (Thales Theorem)
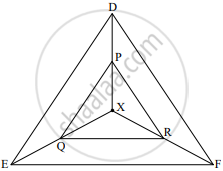
In the given figure, X is any point in the interior of the triangle. Point X is joined to the vertices of triangle. seg PQ || seg DE, seg QR || seg EF. Complete the activity and prove that seg PR || seg DF.
Proof:
In ΔXDE, PQ || DE ......(Given)
∴ `"XP"/"PD" = square/"QE"` ......(Basic proportionality theorem)…(i)
In ΔXEF, QR || EF ......(Given)
∴ `"XQ"/square = "XR"/square` ..........(`square`)....(ii)
∴ `"XP"/"PD" = square/square` ......[From (i) and (ii)]
∴ seg PR || seg DF ......(By converse of basic proportionality theorem
Concept: Converse of Basic Proportionality Theorem
In ΔABC, PQ is a line segment intersecting AB at P and AC at Q such that seg PQ || seg BC. If PQ divides ΔABC into two equal parts having equal areas, find `"BP"/"AB"`.
Concept: Areas of Similar Triangles
If ∆ABC ~ ∆PQR and AB : PQ = 3 : 4 then A(∆ABC) : A(∆PQR) = ?
Concept: Areas of Similar Triangles
In fig, seg DE || sec BC, identify the correct statement.
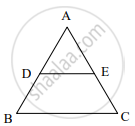
Concept: Basic Proportionality Theorem (Thales Theorem)
In fig., line BC || line DE, AB = 2, BD = 3, AC = 4 and CE = x, then find the value of x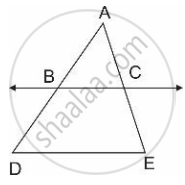
Concept: Basic Proportionality Theorem (Thales Theorem)
ΔPQR ~ ΔSUV. Write pairs of congruent angles
Concept: Similarity of Triangles
Write the test of similarity for triangles given in figure.
Concept: Similarity of Triangles
In fig. BP ⊥ AC, CQ ⊥ AB, A−P−C, and A−Q−B then show that ΔAPB and ΔAQC are similar.
In ΔAPB and ΔAQC
∠APB = [ ]° ......(i)
∠AQC = [ ]° ......(ii)
∠APB ≅ ∠AQC .....[From (i) and (ii)]
∠PAB ≅ ∠QAC .....[______]
ΔAPB ~ ΔAQC .....[______]
Concept: Similarity of Triangles
From fig., seg PQ || side BC, AP = x + 3, PB = x – 3, AQ = x + 5, QC = x – 2, then complete the activity to find the value of x.
In ΔPQB, PQ || side BC
`"AP"/"PB" = "AQ"/(["______"])` ...[______]
`(x + 3)/(x - 3) = (x + 5)/(["______"])`
(x + 3) [______] = (x + 5)(x – 3)
x2 + x – [______] = x2 + 2x – 15
x = [______]
Concept: Basic Proportionality Theorem (Thales Theorem)
In ΔABC, B − D − C and BD = 7, BC = 20, then find the following ratio.
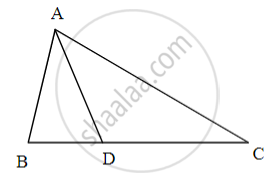
(i) `"A(ΔABD)"/"A(ΔADC)"`
(ii) `"A(ΔABD)"/"A(ΔABC)"`
(iii) `"A(ΔADC)"/"A(ΔABC)"`
Concept: Properties of Ratios of Areas of Two Triangles
In given fig., quadrilateral PQRS, side PQ || side SR, AR = 5 AP, then prove that, SR = 5PQ
Concept: Similarity of Triangles
In Quadrilateral ABCD, side AD || BC, diagonal AC and BD intersect in point P, then prove that `"AP"/"PD" = "PC"/"BP"`
Concept: Similarity of Triangles
Areas of two similar triangles are equal then prove that triangles are congruent
Concept: Similarity of Triangles
In fig., PS = 2, SQ = 6, QR = 5, PT = x and TR = y. Then find the pair of value of x and y such that ST || side QR.
Concept: Basic Proportionality Theorem (Thales Theorem)
If ΔABC ∼ ΔDEF and ∠A = 48°, then ∠D = ______.
Concept: Similarity of Triangles

In the above figure, seg AC and seg BD intersect each other in point P. If `("AP")/("CP") = ("BP")/("DP")`, then complete the following activity to prove ΔABP ∼ ΔCDP.
Activity: In ΔABP and ΔCDP
`("AP")/("CP") = ("BP")/("DP")` ......`square`
∴ ∠APB ≅ `square` ......Vertically opposite angles
∴ `square` ∼ ΔCDP ....... `square` test of similarity.
Concept: Criteria for Similarity of Triangles
