Advertisements
Advertisements
Question
A bucket has top and bottom diameter of 40 cm and 20 cm respectively. Find the volume of the bucket if its depth is 12 cm. Also, find the cost of tin sheet used for making the bucket at the rate of Rs. 1.20 per dm2 . (Use π = 3.14)
Solution
The radii of the top and bottom circles are r1 = 20 cm and r2 = 10 cm respectively. The height of the bucket is h= 12 cm. Therefore, the volume of the bucket is
`V=1/3pi(r_1^2+r_1r_2+r_2^2)xxh`
`=1/3pi(20^2+20xx10+10^2)xx12`
`=1/3xx22/7xx700xx12`
= 8800 cm3
The slant height of the bucket is
`l=sqrt((r_1-r_2)^2+h^2)`
`=sqrt((20+10)^2+12^2)`
`=sqrt(244)`
`=2sqrt(61) cm`
The total surface area of the bucket is
`=pi(r_1-r_2)xxl+pir_2^2`
`=22/7xx(20+10)xx2sqrt(61)+22/7xx10^2`
`=(1320sqrt(61)+2200)/7xxcm^2`
`=(1320sqrt(61)+2200)/7xx100 dm^2`
The total cost of tin sheet used for making the bucket is
`=1.20xx((1320sqrt(61)+2200)/(7xx100))`
= 21.40
APPEARS IN
RELATED QUESTIONS
The largest possible sphere is carved out of a wooden solid cube of side 7 em. Find the volume of the wood left. (Use\[\pi = \frac{22}{7}\]).
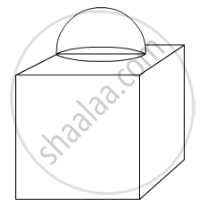
In Fig. 5, is a decorative block, made up two solids – a cube and a hemisphere. The base of the block is a cube of side 6 cm and the hemisphere fixed on the top has diameter of 3.5 cm. Find the total surface area of the bock `(Use pi=22/7)`
A tent is in the shape of a cylinder surmounted by a conical top. If the height and diameter of the cylindrical part are 2.1 m and 4 m respectively, and the slant height of the top is 2.8 m, find the area of the canvas used for making the tent. Also, find the cost of the canvas of the tent at the rate of Rs 500 per m2.
(Note that the base of the tent will not be covered with canvas.) [Use `pi = 22/7`]
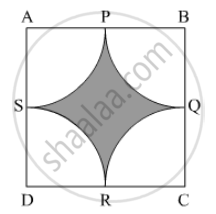
Find the area of the shaded region in Fig. 3, where arcs drawn with centres A, B, C and D intersect in pairs at mid-points P, Q, R and S of the sides AB, BC, CD and DA respectively of a square ABCD of side 12 cm. [Use π = 3.14]
Prove that the surface area of a sphere is equal to the curved surface area of the circumference cylinder__?
A tent consists of a frustum of a cone capped by a cone. If the radii of the ends of the frustum be 13 m and 7 m , the height of the frustum be 8 m and the slant height of the conical cap be 12 m, find the canvas required for the tent. (Take : π = 22/7)
A solid sphere of radius 'r' is melted and recast into a hollow cylinder of uniform thickness. If the external radius of the base of the cylinder is 4 cm, its height 24 cm and thickness 2 cm, find the value of 'r'.
From a solid cube of side 7 cm , a conical cavity of height 7 cm and radius 3 cm is hollowed out . Find the volume of the remaining solid.
Two cubes each of volume 27 cm3 are joined end to end to form a solid. Find the surface area of the resulting cuboid.
Five identical cubes, each of edge 5 cm, are placed adjacent to each other. Find the volume of the resulting cuboid.
