Advertisements
Advertisements
Question
A cell supplies a current of 0.6 A through a 2Ω coil and a current of 0.3 A through on 8Ω coil. Calculate the e.m.f and internal resistance of the cell.
Solution
Given: I1 = 0.6 A, R1 = 2 Ω and I2 = 0.3 A, R2 = 8 Ω
Let the e.m.f. of the cell is E and its internal resistance is r.
From I = `"E"/("R + r")`
0.6 = `"E"/("2 + r")` and `0.3 = "E"/("8 + r")`
∴ E = 0.6 (2 + r) = 1.2 + 0.6 r and
E = 0.3 (8 + r) = 2.4 + 0.3r
Thus, 1.2 + 0.6r = 2.4 + 0.3r
or 0.6r - 0.3r = 2.4 - 1.2
Or 0.3 r = 1.2 or
r = `1.2/0.3 = 4 Omega`.
and E = 1.2 + 0.6 × 4 = 1.2 + 2.4 = 3.6 V
APPEARS IN
RELATED QUESTIONS
A cell of Emf 2 V and internal resistance 1.2 Ω is connected with an ammeter of resistance 0.8 Ω and two resistors of 4.5 Ω and 9 Ω as shown in the diagram below:

1) What would be the reading on the Ammeter?
2) What is the potential difference across the terminals of the cell?
A cell of e.m.f ε and internal resistance r is used to send current to an external resistance R. Write expressions for
- the total resistance of circuit.
- the current drawn from the cell.
- the p.d. across the cell.
- voltage drop inside the cell.
A cell is used to send current to an external circuit.
- How does the voltage across its terminals compare with its e.m.f.?
- Under what condition is the e.m.f. of a cell equal to its terminal voltage?
A battery of e.m.f 3.0 V supplies current through a circuit in which the resistance can be changed.
A high resistance voltmeter is connected across the battery. When the current is 1.5 A, the voltmeter reads 2.7 V. Find the internal resistance of the battery.
Four cells, each of e.m.f. 1.5 V and internal resistance 2.0 ohms are connected in parallel. The battery of cells is connected to an external resistance of 2.5 ohms. Calculate:
(i) The total resistance of the circuit.
(ii) The current flowing in the external circuit.
(iii) The drop in potential across-the terminals of the cells.
Define the e.m.f. (E) of a cell and the potential difference (V) of a resistor R in terms of the work done in moving a unit charge. State the relation between these two works and the work done in moving a unit charge through a cell connected across the resistor. Take the internal resistance of the cell as ‘r’. Hence obtain an expression for the current i in the circuit.
A battery of 4 cell, each of e.m.f. 1.5 volt and internal resistance 0.5 Ω is connected to three resistances as shown in the figure. Calculate:
(i) The total resistance of the circuit.
(ii) The current through the cell.
(iii) The current through each resistance.
(iv) The p.d. across each resistance.
(a) Calculate the total resistance across AB.
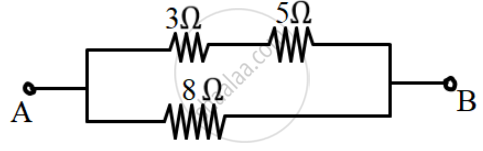
(b) If a cell of e.m.f 2.4 V with negligible internal resistance is connected across AB then calculate the current drawn from the cell.
Study the diagram:
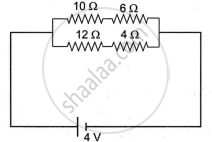
- Calculate the total resistance of the circuit.
- Calculate the current drawn from the cell.
- State whether the current through 10 Ω resistor is greater than, less than or equal to the current through the 12 Ω resistor.
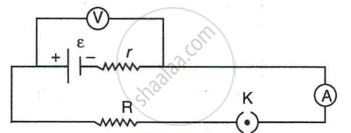
The diagram in Figure shows a cell of e.m.f. ε = 4 volt and internal resistance r = 2 ohm connected to an external resistance R = 8 ohm. The ammeter A measures the current in the circuit and the voltmeter V measures the terminal voltage across the cell. What will be the readings of the ammeter and voltmeter when
- the key K is open, and
- the key K is closed