Question

A chord of a circle of radius 14 cm subtends an angle of 90° at the centre. Find the area of the corresponding minor and major segments of the circle.

Answer 1

Given that,

Radius of circle, r = 14 cm

Angle subtended by chord, θ = 90°

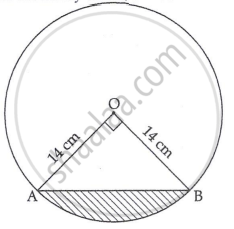
We have,

Area of minor sector = `(pir^2)/(360^circ)`

= `22/7 xx 14 xx 14 xx (90^circ)/(360^circ)`

= 154 cm²

Area of `triangle`AOB = `1/2 xx 14 xx 14` = 98 cm²

Now,

Area of minor segment = Area of minor sector − Area of `triangle`AOB

Area of minor segment = 154 − 98 = 56 cm²

Also,

Area of major segment = Area of circle − Area of minor sector

Area of major segment = πr² − 56

= `22/7 xx 14 xx 14 − 56`

= 616 − 56

= 560 cm²

So, the area of major segment = 560 cm²