Advertisements
Advertisements
Question
A circus tent is cylindrical to a height of 3 m and conical above it. If its base radius is 52.5 m and the slant height of the conical portion is 53 m, find the area of canvas needed to make the tent.
Solution
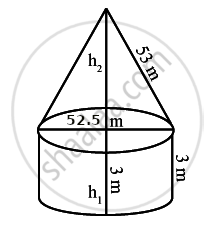
Given,
Height of cylinder (h) = 3 m
Base radius of cylinder (r) = 52.5 m
Slant height of cone (l) = 53 m
Base radius of cone = Base radius of cylinder (r) = 52.5 m
∴ Area of canvas needed = Curved surface area of the cylindrical portion + Curved surface area of the conical portion
∴ Area of canvas needed = πrl + 2πrh
∴ Area of canvas needed = πr(l + 2h)
∴ Area of canvas needed = (`22/7` × 52.5)(53 + 2 × 3)
∴ Area of canvas needed = `22/7` × 52.5 × 59
∴ Area of canvas needed = 9735 m2
APPEARS IN
RELATED QUESTIONS
A cylindrical vessel of radius 4 cm contains water. A solid sphere of radius 3 cm is lowered into the water until it is completely immersed. The water level in the vessel will rise by
A vessel is in the form of a hemispherical bowl surmounted by a hollow cylinder. The diameter of the hemisphere is 21 cm and the total height of the vessel is 14.5 cm. Find its capacity.
A hemispherical bowl of internal radius 9 cm is full of water. Its contents are emptied into a cylindrical vessel of internal radius 6 cm. Find the height of water in the cylindrical vessel.
The total surface area of a cube is 864 cm2. Its volume is
The curved surface are of a cylinder is 1760 cm2 and its base radius is 14 cm. The height of the cylinder is
A cube whose edge is 20 cm long, has circles on each of its faces painted black. What is the total area of the unpainted surface of the cube if the circles are of the largest possible areas?
A sphere and a cube have equal surface areas. The ratio of the volume of the sphere to that of cube is ______.
The capacity of a cylindrical vessel with a hemispherical portion raised upward at the bottom as shown in the figure is `(pir^2)/3 [3h - 2r]`.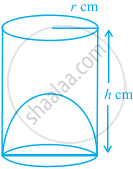
A bicycle wheel makes 500 revolutions in moving 1 km. Find the diameter of the wheel.
A cylindrical tank has a radius of 154 cm. It is filled with water to a height of 3 m. If water to a height of 4.5 m is poured into it, what will be the increase in the volume of water in kl?
