Advertisements
Advertisements
Question
A graph of x versus t is shown in figure. Choose correct alternatives from below.
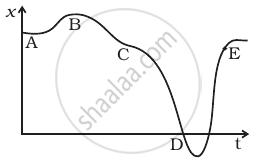
- The particle was released from rest at t = 0.
- At B, the acceleration a > 0.
- At C, the velocity and the acceleration vanish.
- Average velocity for the motion between A and D is positive.
- The speed at D exceeds that at E.
Solution
a, c and e
Explanation:
We know that velocity v = dx/dt and the slope of x-t graph gives ‘us velocity. This implies slope = dx/dt for the graph.
As per the diagram, at point A the graph is parallel to time axis, hence dx
v = dx/dt = 0. As the starting point is A, hence we can say that the particle is starting from rest. Thus option (a) is correct.
At C, the graph changes slope, hence velocity also changes.
As the graph at C is almost parallel to the time axis, hence we can say that velocity vanishes. Hence option (c) is correct.
As the direction of acceleration changes, hence we can say that it may be zero in between.
From the graph, it is clear that | slope at D| > | slope at E |
Hence, the speed at D will be more than at E. Hence option (e) is correct.
Important point: Here, a negative slope does not mean less value. It represents the change in direction of velocity.
APPEARS IN
RELATED QUESTIONS
Read the statement below carefully and state, with reason and example, if it is true or false:
A particle in one-dimensional motion with constant speed must have zero acceleration.
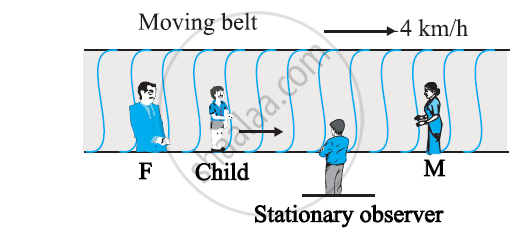
On a long horizontally moving belt (Fig. 3.26), a child runs to and fro with a speed 9 km h–1 (with respect to the belt) between his father and mother located 50 m apart on the moving belt. The belt moves with a speed of 4 km h–1. For an observer on a stationary platform outside, what is the
(a) speed of the child running in the direction of motion of the belt ?.
(b) speed of the child running opposite to the direction of motion of the belt ?
(c) time taken by the child in (a) and (b) ?
Which of the answers alter if motion is viewed by one of the parents?
If a particle is accelerating, it is either speeding up or speeding down. Do you agree with this statement?
Give example where the velocity is opposite in direction to the acceleration.
Give example where the velocity is perpendicular to the acceleration.
A stone is released from an elevator going up with an acceleration a. The acceleration of the stone after the release is
The accelerations of a particle as seen from two frames S1 and S2 have equal magnitude 4 m/s2.
An object having a velocity 4.0 m/s is accelerated at the rate of 1.2 m/s2 for 5.0 s. Find the distance travelled during the period of acceleration.
The displacement of particle is given by x = `2/lambda (1 - e^(-lambdat))`, the acceleration of particle at 2s when λ = 2s–1 will be (Take `l`n 0.018 = – 4)
A lift is coming from 8th floor and is just about to reach 4th floor. Taking ground floor as origin and positive direction upwards for all quantities, which one of the following is correct?
