Advertisements
Advertisements
Question
A man travelled two fifth of his journey by train, one-third by bus, one-fourth by car and the remaining 3 km on foot. What is the length of his total journey?
Solution
Let his total journey length be x.
∴ Then, travelled by train = `2/5x`,
Travelled by bus = `1/3x` and travelled by car = `1/4x`
∴ Total journey travelled by train, bus and car = `2/5x + 1/3x + 1/4x`
= `(12 xx 2x + 20 xx x + 15 xx x)/60` ......[∵ LCM = of 5, 3 and 4 = 60]
= `(24x + 20x + 15x)/60`
= `(59x)/60`
∴ Remaining journey = `x/1 - (59x)/60`
= `(60x - 59x)/60`
= `x/60`
According to the question, the remaining journey is 3 km.
∴ `x/60` = 3
⇒ x = 3 × 60 = 180 km ......[By cross-multiplication]
Hence, the length of his total journey is 180 km.
APPEARS IN
RELATED QUESTIONS
The teacher tells the class that the highest marks obtained by a student in her class is twice the lowest marks plus 7. The highest score is 87. What is the lowest score?
In a test Abha gets twice the marks as that of Palak. Two times Abha's marks and three times Palak's marks make 280.
The equation formed is ______.
In a test Abha gets twice the marks as that of Palak. Two times Abha's marks and three times Palak's marks make 280.
Marks obtained by Abha are ______.
In a bag there are 5 and 2 rupee coins. If they are equal in number and their worth is ₹ 70, then
The equation formed is ______.
A number divided by 2 and then increased by 5 is 9.
In a family, the consumption of wheat is 4 times that of rice. The total consumption of the two cereals is 80 kg. Find the quantities of rice and wheat consumed in the family.
Anamika thought of a number. She multiplied it by 2, added 5 to the product and obtained 17 as the result. What is the number she had thought of?
In a class of 60 students, the number of girls is one-third the number of boys. Find the number of girls and boys in the class.
Radha got ₹ 17,480 as her monthly salary and over-time. Her salary exceeds the over-time by ₹ 10,000. What is her monthly salary?
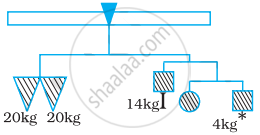
The given figure represents a weighing balance. The weights of some objects in the balance are given. Find the weight of each square and the circle.