Advertisements
Advertisements
Question
A particle having mass 10 g oscillates according to the equation x = (2.0 cm) sin [(100 s−1)t + π/6]. Find (a) the amplitude, the time period and the spring constant. (c) the position, the velocity and the acceleration at t = 0.
Solution
Given:
Equation of motion of the particle executing S.H.M ,
\[x = \left( 2 . 0 cm \right) \sin \left[ \left( 100 s^{- 1} \right)t + \frac{\pi}{6} \right]\]
\[\text { Mass of the particle} , m = 10 g\]...(1)
General equation of the particle is given by,
(a) Amplitude, A is 2 cm.
Angular frequency, ω is 100 s−1.
\[\text { Time period is calculated as }, \]
\[ T = \frac{2\pi}{\omega} = \frac{2\pi}{100} = \frac{\pi}{50}s\]
\[ = 0 . 063 s\]
Also, we know -
\[T = 2\pi\sqrt{\frac{m}{k}}\]
\[\text { where k is the spring constant }. \]
\[ \Rightarrow T^2 = 4 \pi^2 \frac{m}{k}\]
\[ \Rightarrow k = \frac{4 \pi^2 m}{T^2} = {10}^5 dyne/cm\]
\[ = 100 N/m\]
(b) At t = 0 and x = 2 cm
x = A sin (ωt + ϕ)
Using
\[= 2 \times 100 \cos \left( 0 + \frac{\pi}{6} \right)\]
\[ = 200 \times \frac{\sqrt{3}}{2}\]
\[ = 100\sqrt{3} {\text { cms }}^{- 1} \]
\[ = 1 . 73 {\text {ms}}^{- 1}\]
(c) Acceleration of the particle is given by,
a = \[- \omega^2\]x
= 1002×1 = 10000 cm/s2
APPEARS IN
RELATED QUESTIONS
The maximum speed and acceleration of a particle executing simple harmonic motion are 10 cm/s and 50 cm/s2. Find the position(s) of the particle when the speed is 8 cm/s.
The equation of motion of a particle started at t = 0 is given by x = 5 sin (20t + π/3), where x is in centimetre and t in second. When does the particle
(a) first come to rest
(b) first have zero acceleration
(c) first have maximum speed?
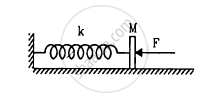
In following figure k = 100 N/m M = 1 kg and F = 10 N.
- Find the compression of the spring in the equilibrium position.
- A sharp blow by some external agent imparts a speed of 2 m/s to the block towards left. Find the sum of the potential energy of the spring and the kinetic energy of the block at this instant.
- Find the time period of the resulting simple harmonic motion.
- Find the amplitude.
- Write the potential energy of the spring when the block is at the left extreme.
- Write the potential energy of the spring when the block is at the right extreme.
The answer of b, e and f are different. Explain why this does not violate the principle of conservation of energy.
Repeat the previous exercise if the angle between each pair of springs is 120° initially.
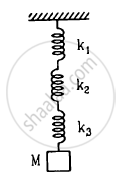
Find the elastic potential energy stored in each spring shown in figure, when the block is in equilibrium. Also find the time period of vertical oscillation of the block.
Solve the previous problem if the pulley has a moment of inertia I about its axis and the string does not slip over it.
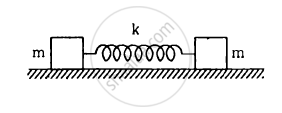
Consider the situation shown in figure . Show that if the blocks are displaced slightly in opposite direction and released, they will execute simple harmonic motion. Calculate the time period.
Find the elastic potential energy stored in each spring shown in figure when the block is in equilibrium. Also find the time period of vertical oscillation of the block.
When the displacement of a particle executing simple harmonic motion is half its amplitude, the ratio of its kinetic energy to potential energy is ______.
A body is executing simple harmonic motion with frequency ‘n’, the frequency of its potential energy is ______.
Motion of an oscillating liquid column in a U-tube is ______.
Displacement versus time curve for a particle executing S.H.M. is shown in figure. Identify the points marked at which (i) velocity of the oscillator is zero, (ii) speed of the oscillator is maximum.

Find the displacement of a simple harmonic oscillator at which its P.E. is half of the maximum energy of the oscillator.
A body of mass m is attached to one end of a massless spring which is suspended vertically from a fixed point. The mass is held in hand so that the spring is neither stretched nor compressed. Suddenly the support of the hand is removed. The lowest position attained by the mass during oscillation is 4 cm below the point, where it was held in hand.
What is the amplitude of oscillation?
An object of mass 0.5 kg is executing a simple Harmonic motion. Its amplitude is 5 cm and the time period (T) is 0.2 s. What will be the potential energy of the object at an instant t = `T/4` s starting from the mean position? Assume that the initial phase of the oscillation is zero.
A particle undergoing simple harmonic motion has time dependent displacement given by x(t) = A sin`(pit)/90`. The ratio of kinetic to the potential energy of this particle at t = 210s will be ______.
The total energy of a particle, executing simple harmonic motion is ______.
where x is the displacement from the mean position, hence total energy is independent of x.
