Advertisements
Advertisements
Question
Motion of an oscillating liquid column in a U-tube is ______.
Options
periodic but not simple harmonic.
non-periodic.
simple harmonic and time period is independent of the density of the liquid.
simple harmonic and time-period is directly proportional to the density of the liquid.
Solution
Motion of an oscillating liquid column in a U-tube is simple harmonic and time period is independent of the density of the liquid.
Explanation:
If the liquid in U-tube is filled to a height of h and the cross-section of the tube is uniform and the liquid is incompressible and non-viscous. Initially, the level of liquid in the two limbs will be at the same height equal to h. If the liquid is pressed by y in one limb, it will rise by y along the length of the tube in the other limb, so the restoring force will be developed by the hydrostatic pressure difference
Here, the hydrostatic pressure provides the restoring force, thus,
F = – V.p.g
= – A.2ypg, where,
A = Area of a cross-section of tube and F ∝ – y,
Thus, it is a simple harmonic motion.
T = `sqrt((2πm("inertia"))/(k("spring"))`
= `sqrt((2πA(2h))/(p2Apg))`
T = `2π sqrt(h/g)`
Thus, the motion is harmonic as the time period is independent of density.
APPEARS IN
RELATED QUESTIONS
A particle is in linear simple harmonic motion between two points, A and B, 10 cm apart. Take the direction from A to B as the positive direction and give the signs of velocity, acceleration and force on the particle when it is
(a) at the end A,
(b) at the end B,
(c) at the mid-point of AB going towards A,
(d) at 2 cm away from B going towards A,
(e) at 3 cm away from A going towards B, and
(f) at 4 cm away from B going towards A.
Consider a particle moving in simple harmonic motion according to the equation x = 2.0 cos (50 πt + tan−1 0.75) where x is in centimetre and t in second. The motion is started at t = 0. (a) When does the particle come to rest for the first time? (b) When does he acceleration have its maximum magnitude for the first time? (c) When does the particle come to rest for the second time ?
The pendulum of a clock is replaced by a spring-mass system with the spring having spring constant 0.1 N/m. What mass should be attached to the spring?
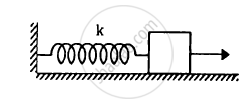
The spring shown in figure is unstretched when a man starts pulling on the cord. The mass of the block is M. If the man exerts a constant force F, find (a) the amplitude and the time period of the motion of the block, (b) the energy stored in the spring when the block passes through the equilibrium position and (c) the kinetic energy of the block at this position.
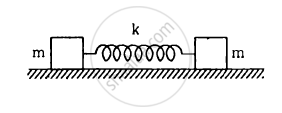
Consider the situation shown in figure . Show that if the blocks are displaced slightly in opposite direction and released, they will execute simple harmonic motion. Calculate the time period.
Discuss in detail the energy in simple harmonic motion.
When the displacement of a particle executing simple harmonic motion is half its amplitude, the ratio of its kinetic energy to potential energy is ______.
A body is executing simple harmonic motion with frequency ‘n’, the frequency of its potential energy is ______.
A body is performing S.H.M. Then its ______.
- average total energy per cycle is equal to its maximum kinetic energy.
- average kinetic energy per cycle is equal to half of its maximum kinetic energy.
- mean velocity over a complete cycle is equal to `2/π` times of its π maximum velocity.
- root mean square velocity is times of its maximum velocity `1/sqrt(2)`.
Draw a graph to show the variation of P.E., K.E. and total energy of a simple harmonic oscillator with displacement.
