Advertisements
Advertisements
प्रश्न
Motion of an oscillating liquid column in a U-tube is ______.
विकल्प
periodic but not simple harmonic.
non-periodic.
simple harmonic and time period is independent of the density of the liquid.
simple harmonic and time-period is directly proportional to the density of the liquid.
उत्तर
Motion of an oscillating liquid column in a U-tube is simple harmonic and time period is independent of the density of the liquid.
Explanation:
If the liquid in U-tube is filled to a height of h and the cross-section of the tube is uniform and the liquid is incompressible and non-viscous. Initially, the level of liquid in the two limbs will be at the same height equal to h. If the liquid is pressed by y in one limb, it will rise by y along the length of the tube in the other limb, so the restoring force will be developed by the hydrostatic pressure difference
Here, the hydrostatic pressure provides the restoring force, thus,
F = – V.p.g
= – A.2ypg, where,
A = Area of a cross-section of tube and F ∝ – y,
Thus, it is a simple harmonic motion.
T = `sqrt((2πm("inertia"))/(k("spring"))`
= `sqrt((2πA(2h))/(p2Apg))`
T = `2π sqrt(h/g)`
Thus, the motion is harmonic as the time period is independent of density.
APPEARS IN
संबंधित प्रश्न
A particle executes simple harmonic motion with an amplitude of 10 cm. At what distance from the mean position are the kinetic and potential energies equal?
The maximum speed and acceleration of a particle executing simple harmonic motion are 10 cm/s and 50 cm/s2. Find the position(s) of the particle when the speed is 8 cm/s.
A block suspended from a vertical spring is in equilibrium. Show that the extension of the spring equals the length of an equivalent simple pendulum, i.e., a pendulum having frequency same as that of the block.
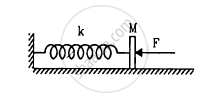
In following figure k = 100 N/m M = 1 kg and F = 10 N.
- Find the compression of the spring in the equilibrium position.
- A sharp blow by some external agent imparts a speed of 2 m/s to the block towards left. Find the sum of the potential energy of the spring and the kinetic energy of the block at this instant.
- Find the time period of the resulting simple harmonic motion.
- Find the amplitude.
- Write the potential energy of the spring when the block is at the left extreme.
- Write the potential energy of the spring when the block is at the right extreme.
The answer of b, e and f are different. Explain why this does not violate the principle of conservation of energy.
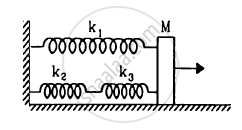
The springs shown in the figure are all unstretched in the beginning when a man starts pulling the block. The man exerts a constant force F on the block. Find the amplitude and the frequency of the motion of the block.
Solve the previous problem if the pulley has a moment of inertia I about its axis and the string does not slip over it.
If a body is executing simple harmonic motion and its current displacements is `sqrt3/2` times the amplitude from its mean position, then the ratio between potential energy and kinetic energy is:
A body is executing simple harmonic motion with frequency ‘n’, the frequency of its potential energy is ______.
A mass of 2 kg is attached to the spring of spring constant 50 Nm–1. The block is pulled to a distance of 5 cm from its equilibrium position at x = 0 on a horizontal frictionless surface from rest at t = 0. Write the expression for its displacement at anytime t.
An object of mass 0.5 kg is executing a simple Harmonic motion. Its amplitude is 5 cm and the time period (T) is 0.2 s. What will be the potential energy of the object at an instant t = `T/4` s starting from the mean position? Assume that the initial phase of the oscillation is zero.
