Advertisements
Advertisements
Question
Discuss in detail the energy in simple harmonic motion.
Solution
Energy in simple harmonic motion:
(a) Expression for Potential Energy: For the simple harmonic motion, the force and the displacement are related by Hooke’s law
`vec"F" = -"k"vec("r")`
Since force is a vector quantity, in three dimensions it has three components.
Further, the force in the above equation is a conservative force field; such a force can be derived from a scalar function which has only one component. In one dimensional case
F = − kx .....................(1)
The work done by the conservative force field is independent of the path. The potential energy U can be calculated from the following expression.
F = `-"dU"/"dx"` ..........(2)
Comparing (1) and (2), we get
`-"dU"/"dx" = -"kx"`
dU = kx dx
This work done by the force F during a small displacement dx stores as potential energy
U(x) = `int_0^"x" "kx′" "dx′" = 1/2["k"("x′")^2]_0^"x" = 1/2"kx"^2` ..........(3)
From equation `ω = sqrt("k"/"m")`, we can substitute the value of force constant k = mω2 in equation (3),
U(x) = `1/2"m"ω^2"x"^2` ...................(4)
where ω is the natural frequency of the oscillating system. For the particle executing simple harmonic motion from equation y = A sin ωt, we get
x = A sin ωt
U(t) = `1/2"m"ω^2"A"^2 sin^2ω"t"` ............(5)
This variation of U is shown in figure.
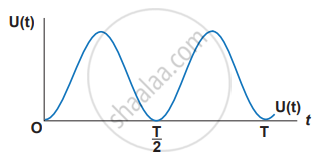
Variation of potential energy with time t
(b) Expression for Kinetic Energy:
Kinetic energy KE = `1/2"mv"_"x"^2 = 1/2"m" ("dx"/"dt")^2` ............(6)
Since the particle is executing simple harmonic motion, from equation y = A sin ωt
x = A sin ωt
Therefore, velocity is
vx = `"dx"/"dt"` = Aω cos ωt ...................(7)
= `"A"ω sqrt(1 - ("x"/"A")^2)`
vx = `ω sqrt("A"^2 - "x"^2)`
Hence, KE = `1/2"mv"_"x"^2 = 1/2"m"ω^2 ("A"^2 - "x"^2)` ........(9)
KE = `1/2"m"ω^2 "A"^2 cos^2 ω"t"` ..............(10)
This variation with time is shown in figure.
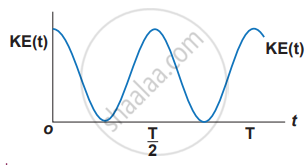
Variation of kinetic energy with time t
(c) Expression for Total Energy: Total energy is the sum of kinetic energy and potential energy
E = KE + U ..........…(11)
E = `1/2"m"ω^2 ("A"^2 - "x"^2) + 1/2 "m"ω^2 "x"^2`
Hence, cancelling x2 term,
E = `1/2"m"ω^2"A"^2` = constant ...............(12)
Alternatively, from equation (5) and equation (10), we get the total energy as
E = `1/2"m"ω^2"A"^2 sin^2 ω"t" + 1/2"m"ω^2"A"^2 cos^2 ω"t"`
= `1/2"m"ω^2"A"^2 (sin^2 ω"t" + cos^2 ω"t")`
From trigonometry identity, (sin2 ωt + cos2 ωt) = 1
E = `1/2"m"ω^2"A"^2` = constant
which gives the law of conservation of total energy. This is depicted in Figure
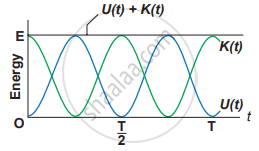
Both kinetic energy and potential energy vary but total energy is constant
Thus the amplitude of a simple harmonic oscillator can be expressed in terms of total energy.
A = `sqrt((2"E")/("m"ω^2)) = sqrt((2"E")/"k")` ..........(13)
APPEARS IN
RELATED QUESTIONS
A particle executes simple harmonic motion with an amplitude of 10 cm. At what distance from the mean position are the kinetic and potential energies equal?
A particle having mass 10 g oscillates according to the equation x = (2.0 cm) sin [(100 s−1)t + π/6]. Find (a) the amplitude, the time period and the spring constant. (c) the position, the velocity and the acceleration at t = 0.
The pendulum of a clock is replaced by a spring-mass system with the spring having spring constant 0.1 N/m. What mass should be attached to the spring?
The block of mass m1 shown in figure is fastened to the spring and the block of mass m2 is placed against it. (a) Find the compression of the spring in the equilibrium position. (b) The blocks are pushed a further distance (2/k) (m1 + m2)g sin θ against the spring and released. Find the position where the two blocks separate. (c) What is the common speed of blocks at the time of separation?
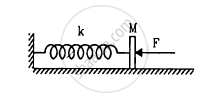
In following figure k = 100 N/m M = 1 kg and F = 10 N.
- Find the compression of the spring in the equilibrium position.
- A sharp blow by some external agent imparts a speed of 2 m/s to the block towards left. Find the sum of the potential energy of the spring and the kinetic energy of the block at this instant.
- Find the time period of the resulting simple harmonic motion.
- Find the amplitude.
- Write the potential energy of the spring when the block is at the left extreme.
- Write the potential energy of the spring when the block is at the right extreme.
The answer of b, e and f are different. Explain why this does not violate the principle of conservation of energy.
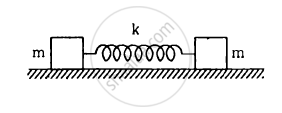
Consider the situation shown in figure . Show that if the blocks are displaced slightly in opposite direction and released, they will execute simple harmonic motion. Calculate the time period.
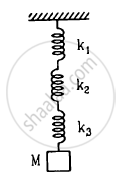
Find the elastic potential energy stored in each spring shown in figure when the block is in equilibrium. Also find the time period of vertical oscillation of the block.
Show that for a particle executing simple harmonic motion.
- the average value of kinetic energy is equal to the average value of potential energy.
- average potential energy = average kinetic energy = `1/2` (total energy)
Hint: average kinetic energy = <kinetic energy> = `1/"T" int_0^"T" ("Kinetic energy") "dt"` and
average potential energy = <potential energy> = `1/"T" int_0^"T" ("Potential energy") "dt"`
When the displacement of a particle executing simple harmonic motion is half its amplitude, the ratio of its kinetic energy to potential energy is ______.
A mass of 2 kg is attached to the spring of spring constant 50 Nm–1. The block is pulled to a distance of 5 cm from its equilibrium position at x = 0 on a horizontal frictionless surface from rest at t = 0. Write the expression for its displacement at anytime t.
