Advertisements
Advertisements
Question
Draw a graph to show the variation of P.E., K.E. and total energy of a simple harmonic oscillator with displacement.
Solution
The potential energy (PE) of a simple harmonic oscillator is = `1/2 kx^2`
= `1/2 mω^2x^2` .....(i)
Where, k = force constant = `mω^2`
When PE is plotted against displacement x, we will obtain a parabola.
When x = 0, PE = 0
When x = ± A, PE = maximum = `1/2 mω^2A^2`
KE of a simple harmonic oscillator = `1/2 mv^2` .....`[∵ v = ωsqrt(A^2 - x^2)]`
= `1/2 m[ωsqrt(A^2 - x^2)]^2`
= `1/2 mω^2(A^2 - x^2)` ......(ii)
This is also a parabola if plotting KE against displacement x.
i.e., KE = 0 at x = ± A
And KE = `1/2 mω^2A^2` at x = 0
Now, the total energy of the simple harmonic oscillator = PE + KE .......[Using equations (i) and (ii)]
= `1/2 mω^2x^2 + 1/2 mω^2 (A^2 - x^2)`
= `1/2 mω^2x^2 + 1/2 mω^2A^2 - 1/2 mω^2x^2`
TE = `1/2 mω^2A^2`
Which is constant and does not depend on x.
Plotting under the above guidelines KE, PE and TE versus displacement x-graph as follows

APPEARS IN
RELATED QUESTIONS
A particle is in linear simple harmonic motion between two points, A and B, 10 cm apart. Take the direction from A to B as the positive direction and give the signs of velocity, acceleration and force on the particle when it is
(a) at the end A,
(b) at the end B,
(c) at the mid-point of AB going towards A,
(d) at 2 cm away from B going towards A,
(e) at 3 cm away from A going towards B, and
(f) at 4 cm away from B going towards A.
Consider a particle moving in simple harmonic motion according to the equation x = 2.0 cos (50 πt + tan−1 0.75) where x is in centimetre and t in second. The motion is started at t = 0. (a) When does the particle come to rest for the first time? (b) When does he acceleration have its maximum magnitude for the first time? (c) When does the particle come to rest for the second time ?
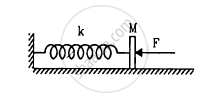
In following figure k = 100 N/m M = 1 kg and F = 10 N.
- Find the compression of the spring in the equilibrium position.
- A sharp blow by some external agent imparts a speed of 2 m/s to the block towards left. Find the sum of the potential energy of the spring and the kinetic energy of the block at this instant.
- Find the time period of the resulting simple harmonic motion.
- Find the amplitude.
- Write the potential energy of the spring when the block is at the left extreme.
- Write the potential energy of the spring when the block is at the right extreme.
The answer of b, e and f are different. Explain why this does not violate the principle of conservation of energy.
Find the elastic potential energy stored in each spring shown in figure, when the block is in equilibrium. Also find the time period of vertical oscillation of the block.
Discuss in detail the energy in simple harmonic motion.
Show that for a particle executing simple harmonic motion.
- the average value of kinetic energy is equal to the average value of potential energy.
- average potential energy = average kinetic energy = `1/2` (total energy)
Hint: average kinetic energy = <kinetic energy> = `1/"T" int_0^"T" ("Kinetic energy") "dt"` and
average potential energy = <potential energy> = `1/"T" int_0^"T" ("Potential energy") "dt"`
When a particle executing S.H.M oscillates with a frequency v, then the kinetic energy of the particle?
A body is executing simple harmonic motion with frequency ‘n’, the frequency of its potential energy is ______.
A mass of 2 kg is attached to the spring of spring constant 50 Nm–1. The block is pulled to a distance of 5 cm from its equilibrium position at x = 0 on a horizontal frictionless surface from rest at t = 0. Write the expression for its displacement at anytime t.
A particle undergoing simple harmonic motion has time dependent displacement given by x(t) = A sin`(pit)/90`. The ratio of kinetic to the potential energy of this particle at t = 210s will be ______.
