Advertisements
Advertisements
Question
A particle performing linear S.H.M. of period 2π seconds about the mean position O is observed to have a speed of `"b" sqrt3` m/s, when at a distance b (metre) from O. If the particle is moving away from O at that instant, find the time required by the particle, to travel a further distance b.
Solution
Data:
T = 2πs, v = `"b" sqrt3` m/s at x = b
∴ ω = `(2pi)/"T" = (2pi)/(2pi)` = 1 rad/s
v = `ω sqrt("A"^2 - "x"^2)`
∴ At x = b,
`"b" sqrt3 = (1) sqrt("A"^2 - "b"^2)`
∴ 3b2 = A2 − b2
∴ A = 2b
∴ Assuming the particle starts from the mean position, its displacement is given by x = A sin ωt = 2b sin t
If the particle is at x = b at t = t1,
b = 2b sint1
∴ t1 = sin−1 `1/2` = `pi/6`s
Also, with period T = 2πs, on travelling a further distance b the particle will reach the positive extremity at time t2 = `π/2`s.
∴ The time taken to travel a further distance b from x = b is
t2 − t1 = `pi/2 - pi/6`
= `(3pi - pi)/6`
= `(2pi)/6`
= `pi/3`s
RELATED QUESTIONS
Choose the correct option:
A particle performs linear S.H.M. starting from the mean position. Its amplitude is A and time period is T. At the instance when its speed is half the maximum speed, its displacement x is ______.
The displacement of an oscillating particle is given by x = a sin ω t + b cos ω t where a, b and ω are constants. Prove that the particle performs a linear S.H.M. with amplitude A = `sqrt("a"^2 + "b"^2)`.
A wooden block of mass m is kept on a piston that can perform vertical vibrations of adjustable frequency and amplitude. During vibrations, we don’t want the block to leave the contact with the piston. How much maximum frequency is possible if the amplitude of vibrations is restricted to 25 cm? In this case, how much is the energy per unit mass of the block? (g ≈ π2 ≈ 10 m/s-2)
The period of oscillation of the simple pendulum increases by 20 %, when its length is increased by 44 cm. find its initial length.
What is meant by periodic motion? Give any two examples, for periodic motion.
What is meant by non-periodic motion? Give any two examples, for non-periodic motion.
What is meant by the force constant of a spring?
A block of mass m attached to one end of the vertical spring produces extension x. If the block is pulled and released, the periodic time of oscillation is
A body of mass 64 g is made to oscillate turn by turn on two different springs A and B. Spring A and B has force constant 4 `"N"/"m"` and `16 "N"/"m"` respectively. If T1 and T2 are period of m oscillations of springs A and B respectively, then `("T"_1 + "T"_2)/("T"_1 - "T"_2)` will be ______.
The displacement of the particle executing linear S.H.M. is x = 0.25 Sill ( 11 l + 0.5) m. The period of S.H.M. is ______.
(`pi = 22/7` )
The ratio of frequencies of oscillations of two simple pendulums is 3: 4 then their lengths are in the ratio ______.
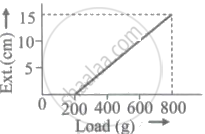
The pointer reading v/s load graph for a spring balance is as given in the figure. The spring constant is ______.
The path length of oscillation of simple pendulum of length 1 meter is 16 cm. Its maximum velocity is ______. (g = π2 m/s2)
In an oscillator, for sustained oscillations, Barkhausen criterion is Aβ equal to ______.
(A = voltage gain without feedback and β feedback factor)
A particle moves in SHM such that its acceleration is a = -px, where x is the displacement of a particle from the equilibrium position and p is a constant. The period of oscillation is ______.
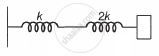
If KS and KP respectively are effective spring constants in series and parallel configurations of springs as shown in the figure. Find `K_S/K_P`.
 |
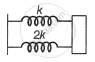 |
| (i) | (ii) |
A block of mass 40 grams is suspended from an ideal spring and set into vertical oscillations. Find the mass to be added on the spring such that the period of oscillation increases by 25%.
