Advertisements
Online Mock Tests
Chapters
2: Mechanical Properties of Fluids
3: Kinetic Theory of Gases and Radiation
4: Thermodynamics
▶ 5: Oscillations
6: Superposition of Waves
7: Wave Optics
8: Electrostatics
9: Current Electricity
10: Magnetic Fields due to Electric Current
11: Magnetic Materials
12: Electromagnetic induction
13: AC Circuits
14: Dual Nature of Radiation and Matter
15: Structure of Atoms and Nuclei
16: Semiconductor Devices
![Balbharati solutions for Physics [English] 12 Standard HSC Maharashtra State Board chapter 5 - Oscillations Balbharati solutions for Physics [English] 12 Standard HSC Maharashtra State Board chapter 5 - Oscillations - Shaalaa.com](/images/physics-english-12-standard-hsc-maharashtra-state-board_6:60ec3c6386c44147a5060ebeda74b4c4.JPG)
Advertisements
Solutions for Chapter 5: Oscillations
Below listed, you can find solutions for Chapter 5 of Maharashtra State Board Balbharati for Physics [English] 12 Standard HSC Maharashtra State Board.
Balbharati solutions for Physics [English] 12 Standard HSC Maharashtra State Board 5 Oscillations Exercises [Pages 129 - 130]
Choose the correct option:
A particle performs linear S.H.M. starting from the mean position. Its amplitude is A and time period is T. At the instance when its speed is half the maximum speed, its displacement x is ______.
`sqrt3/2 A`
`2/sqrt3 A`
`A/2`
`1/sqrt2A`
Choose the correct option:
A body of mass 1 kg is performing linear S.H.M. Its displacement x (cm) at t(second) is given by x = 6 sin `(100t + π/4)`. Maximum kinetic energy of the body is ______.
36J
9J
27J
18J
Choose the correct option:
The length of second's pendulum on the surface of earth is nearly 1 m. Its length on the surface of moon should be [Given: acceleration due to gravity (g) on moon is `1/6` th of that on the earth’s surface]
`1/6`m
6 m
`1/36` m
`1/sqrt6`m
Choose the correct option:
Two identical springs of constant k are connected, first in series and then in parallel. A metal block of mass m is suspended from their combination. The ratio of their frequencies of vertical oscillations will be in a ratio
1:4
1:2
2:1
4:1
Choose the correct option:
The graph shows variation of displacement of a particle performing S.H.M. with time t. Which of the following statements is correct from the graph?
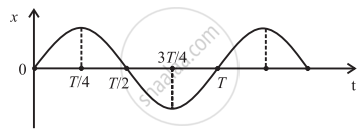
The acceleration is maximum at time T.
The force is maximum at time `(3T)/4`.
The velocity is zero at time `T/2`.
The kinetic energy is equal to total energy at a time `T/4`.
Define linear simple harmonic motion.
Answer in brief.
Using differential equations of linear S.H.M, obtain the expression for (a) velocity in S.H.M., (b) acceleration in S.H.M.
Obtain the expression for the period of a simple pendulum performing S.H.M.
Answer in brief.
State the law of simple pendulum.
Prove that under certain conditions a magnet vibrating in a uniform magnetic field performs angular S.H.M.
Obtain the expression for the period of a magnet vibrating in a uniform magnetic field and performing S.H.M.
Show that a linear S.H.M. is the projection of a U.C.M. along any of its diameter.
Draw graphs of displacement, velocity, and acceleration against phase angle, for a particle performing linear S.H.M. from
- the mean position
- the positive extreme position.
Deduce your conclusions from the graph.
Deduce the expressions for the kinetic energy and potential energy of a particle executing S.H.M. Hence obtain the expression for the total energy of a particle performing S.H.M and show that the total energy is conserved. State the factors on which total energy depends.
Answer in brief:
Derive an expression for the period of motion of a simple pendulum. On which factors does it depend?
At what distance from the mean position is the speed of a particle performing S.H.M. half its maximum speed. Given the path length of S.H.M. = 10 cm.
In SI units, the differential equation of an S.H.M. is `("d"^2"x")/("dt"^2)` = − 36x. Find its frequency and period.
A needle of a sewing machine moves along a path of amplitude 4 cm with a frequency of 5 Hz. Find its acceleration `(1/30)` s after it has crossed the mean position.
Potential energy of a particle performing linear S.H.M. is 0.1 π2x2 joule. If the mass of the particle is 20 g, find the frequency of S.H.M.
The total energy of a body of mass 2 kg performing S.H.M. is 40 J. Find its speed while crossing the center of the path.
A simple pendulum performs S.H.M. of period 4 seconds. How much time after crossing the mean position, will the displacement of the bob be one-third of its amplitude.
A simple pendulum of length 100 cm performs S.H.M. Find the restoring force acting on its bob of mass 50 g when the displacement from the mean position is 3 cm.
Find the change in length of a second’s pendulum, if the acceleration due to gravity at the place changes from 9.75 m/s2 to 9.8 m/s2.
At what distance from the mean position is the kinetic energy of a particle performing S.H.M. of amplitude 8 cm, three times its potential energy?
A particle performing linear S.H.M. of period 2π seconds about the mean position O is observed to have a speed of `"b" sqrt3` m/s, when at a distance b (metre) from O. If the particle is moving away from O at that instant, find the time required by the particle, to travel a further distance b.
The period of oscillation of a body of mass m1 suspended from a light spring is T. When a body of mass m2 is tied to the first body and the system is made to oscillate, the period is 2T. Compare the masses m1 and m2
The displacement of an oscillating particle is given by x = a sin ω t + b cos ω t where a, b and ω are constants. Prove that the particle performs a linear S.H.M. with amplitude A = `sqrt("a"^2 + "b"^2)`.
Two parallel S.H.M.s represented by `"x"_1 = 5 sin(4π"t" + π//3)` cm and `"x"_2 = 3sin (4π"t" + π//4)` cm are superposed on a particle. Determine the amplitude and epoch of the resultant S.H.M.
A 20 cm wide thin circular disc of mass 200 g is suspended to rigid support from a thin metallic string. By holding the rim of the disc, the string is twisted through 60° and released. It now performs angular oscillations of period 1 second. Calculate the maximum restoring torque generated in the string under undamped conditions. (π3 ≈ 31)
Find the number of oscillations performed per minute by a magnet is vibrating in the plane of a uniform field of 1.6 × 10-5 Wb/m2. The magnet has a moment of inertia 3 × 10-6 kg/m2 and magnetic moment 3 A m2.
A wooden block of mass m is kept on a piston that can perform vertical vibrations of adjustable frequency and amplitude. During vibrations, we don’t want the block to leave the contact with the piston. How much maximum frequency is possible if the amplitude of vibrations is restricted to 25 cm? In this case, how much is the energy per unit mass of the block? (g ≈ π2 ≈ 10 m/s-2)
Solutions for 5: Oscillations
![Balbharati solutions for Physics [English] 12 Standard HSC Maharashtra State Board chapter 5 - Oscillations Balbharati solutions for Physics [English] 12 Standard HSC Maharashtra State Board chapter 5 - Oscillations - Shaalaa.com](/images/physics-english-12-standard-hsc-maharashtra-state-board_6:60ec3c6386c44147a5060ebeda74b4c4.JPG)
Balbharati solutions for Physics [English] 12 Standard HSC Maharashtra State Board chapter 5 - Oscillations
Shaalaa.com has the Maharashtra State Board Mathematics Physics [English] 12 Standard HSC Maharashtra State Board Maharashtra State Board solutions in a manner that help students grasp basic concepts better and faster. The detailed, step-by-step solutions will help you understand the concepts better and clarify any confusion. Balbharati solutions for Mathematics Physics [English] 12 Standard HSC Maharashtra State Board Maharashtra State Board 5 (Oscillations) include all questions with answers and detailed explanations. This will clear students' doubts about questions and improve their application skills while preparing for board exams.
Further, we at Shaalaa.com provide such solutions so students can prepare for written exams. Balbharati textbook solutions can be a core help for self-study and provide excellent self-help guidance for students.
Concepts covered in Physics [English] 12 Standard HSC Maharashtra State Board chapter 5 Oscillations are Oscillations, Explanation of Periodic Motion, Linear Simple Harmonic Motion (S.H.M.), Differential Equation of Linear S.H.M., Acceleration (a), Velocity (v) and Displacement (x) of S.H.M., Amplitude (A), Period (T) and Frequency (N) of S.H.M., Reference Circle Method, Phase in S.H.M., Graphical Representation of S.H.M., Composition of Two S.H.M.’S Having Same Period and Along Same Line, The Energy of a Particle Performing S.H.M., Simple Pendulum, Angular S.H.M. and It's Differential Equation, Damped Oscillations, Free Oscillations, Forced Oscillations and Resonance Oscillations, Periodic and Oscillatory Motion.
Using Balbharati Physics [English] 12 Standard HSC Maharashtra State Board solutions Oscillations exercise by students is an easy way to prepare for the exams, as they involve solutions arranged chapter-wise and also page-wise. The questions involved in Balbharati Solutions are essential questions that can be asked in the final exam. Maximum Maharashtra State Board Physics [English] 12 Standard HSC Maharashtra State Board students prefer Balbharati Textbook Solutions to score more in exams.
Get the free view of Chapter 5, Oscillations Physics [English] 12 Standard HSC Maharashtra State Board additional questions for Mathematics Physics [English] 12 Standard HSC Maharashtra State Board Maharashtra State Board, and you can use Shaalaa.com to keep it handy for your exam preparation.
