Advertisements
Advertisements
Question
Obtain the expression for the period of a simple pendulum performing S.H.M.
Solution
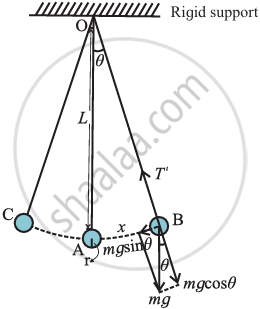
Let m is Mass of the bob.
L is the Length of mass-less string.
A free-body diagram to the forces acting on the bob,
θ − angle made by the string with the vertical.
T is tension along the string
g is the acceleration due to gravity
∴ Restoring force, F = −mg sin θ ...(i)
As θ is very small (θ < 10°), we can write
sin θ ≅ θ° ∴ F ≅ mgθ
From the figure, the small angle `theta = x/L`
∴ `F = -mgx/L` ...(ii)
As m, g and L are constants, F ∝ −x
Thus, for small displacement, the restoring force is directly proportional to the displacement and is oppositely directed.
Hence the bob of a simple pendulum performs linear S.H.M. for small amplitudes. The period T of oscillation of a pendulum from can be given as,
= `(2pi)/omega`
= `(2pi)/sqrt("acceleration per unit displacement")`
Using eq. (ii), `F = -mgx/L`
∴ `a = -gx/L`
∴ `a/x = -g/L = g/L` ...(in magnitude)
Substituting in the expression for T, we get,
`T = 2pi sqrt((L)/(g))` ...(iii)
The equation (iii) gives the expression for the time period of a simple pendulum.
APPEARS IN
RELATED QUESTIONS
Define an ideal simple pendulum.
A simple pendulum performs S.H.M. of period 4 seconds. How much time after crossing the mean position, will the displacement of the bob be one-third of its amplitude.
A simple pendulum of length 100 cm performs S.H.M. Find the restoring force acting on its bob of mass 50 g when the displacement from the mean position is 3 cm.
In a second’s pendulum, the mass of Bob is 50 g. If it is replaced by 100 g mass, then its period will be ______.
The length of a simple pendulum is increased by 44%. The percentage change in its time period is ______
Work done in stretching a wire through 1 mm is 2 J. What amount of work will be done for elongating another wire of same material, with half the length and double the radius of cross-section, by 1 mm?
A simple pendulum has a time period T1 when on the earth's surface and T2 when taken to a height R above the earth's surface, where R is the radius of the earth. The value of T2 / T1 is ______.
The period of simple pendulum is measured as T in a stationary lift. If the lift moves upward with an acceleration of 5 g, the period will ____________.
If a pendulum which gives correct time beats second on ground at a certain place is moved to the top of a tower 320 m high, then the loss of time of the pendulum clock in one day, in second, is ______.
A pendulum performs S.H.M. with period `sqrt3` second in a stationary lift. If lift moves up with acceleration `"g"/3`, the period of pendulum is ______. (g = acceleration due to gravity)
A simple pendulum has length 'L1'. When its amplitude is 'a' cm, its energy is 'E1'. When length is 2L1, its energy is 'E2' Amplitude is same for both the lengths The relation between E1 and E2 is ______.
Two simple pendulums of lengths 1.44 m and 1.96 m start swinging together. After how many vibrations will they again start swinging together?
The length of a seconds pendulum is 1m on the earth. If the mass and diameter of the planet is double than that of the earth, then the length of the second's pendulum on the planet will be ______
A simple pendulum has a length of 2m and a bob of mass of 100 grams. It is whirled in a horizontal plane. If the string breaks under a tension of 10 N, the angle made by the string with vertical is ______
(g = 10 m/s2)
Define second's pendulum.
The bob of a simple pendulum performs SHM with period T in air and with period T1 in water Relation between T and T1 is ______.
(neglect friction due to water, density of the material of the bob is = `9/8xx10^3` kgm3, density of water = 1 gcc-1)
A pendulum bob is swinging in a vertical plane, such that its angular amplitude is less than 90°. At its highest point, the string is cut. Which trajectory is possible for bob afterwards?
Two weights w1 and w2 are suspended from the ends of light string over a smooth fixed pulley. If the pulley is pulled up with acceleration g, the tension in the string will be ______.
A simple pendulum of length 196 cm performs linear SHM of amplitude 8 cm. Find the restoring force on its bob of mass 50 g at an extreme position.
The velocity of bob of a second’s pendulum when it is 6 cm from its mean position and amplitude of 10 cm, is ______.
