Advertisements
Advertisements
Question
Acceleration of a particle executing S.H.M. at its mean position.
Options
Is infinity
Varies
Is maximum
Is zero
Solution
The acceleration of a particle executing S.H.M. at its mean position is zero.
RELATED QUESTIONS
Choose the correct option:
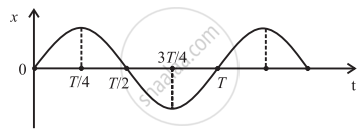
The graph shows variation of displacement of a particle performing S.H.M. with time t. Which of the following statements is correct from the graph?
A particle is performing simple harmonic motion with amplitude A and angular velocity ω. The ratio of maximum velocity to maximum acceleration is ______.
The light of wavelength '`lambda`'. incident on the surface of metal having work function `phi` emits the electrons. The maximum velocity of electrons emitted is ______.
[c = velocity of light, h = Planck's constant, m = mass of electron]
For a particle performing SHM when displacement is x, the potential energy and restoring force acting on it is denoted by E and F, respectively. The relation between x, E and F is ____________.
The displacement of a particle from its mean position (in metre) is given by, y = 0.2 sin(10 πt + 1.5π) cos(10 πt + 1.5π).
The motion of particle is ____________.
A particle is moving along a circular path of radius 6 m with a uniform speed of 8 m/s. The average acceleration when the particle completes one-half of the revolution is ______.
The relation between time and displacement for two particles is given by Y1 = 0.06 sin 27`pi` (0.04t + `phi_1`), y2 = 0.03sin 27`pi`(0.04t + `phi_2`). The ratio of the intensity of the waves produced by the vibrations of the two particles will be ______.
The phase difference between the instantaneous velocity and acceleration of a particle executing S.H.M is ____________.
The distance covered by a particle undergoing SHM in one time period is (amplitude = A) ____________.
A particle executing S.H.M. has amplitude 0.01 m and frequency 60 Hz. The maximum acceleration of the particle is ____________.
A body of mass 5 g is in S.H.M. about a point with amplitude 10 cm. Its maximum velocity is 100 cm/s. Its velocity will be 50 cm/s at a distance of, ____________.
The maximum speed of a particle in S.H.M. is 'V'. The average speed is ______
The length of the second's pendulum is decreased by 0.3 cm when it is shifted from place A to place B. If the acceleration due to gravity at place A is 981 cm/s2, the acceleration due to gravity at place B is ______ (Take π2 = 10)
The bob of a simple pendulum is released at time t = 0 from a position of small angular displacement. Its linear displacement is ______.
(l = length of simple pendulum and g = acceleration due to gravity, A = amplitude of S.H.M.)
The displacement of the particle performing S.H.M. is given by x = 4 sin πt, where x is in cm and t is in second. The time taken by the particle in second to move from the equilibrium position to the position of half the maximum displacement, is ______.
`[sin30^circ=cos60^circ=0.5, cos30^circ=sin60^circ=sqrt3/2]`
The displacement of a particle in S.H.M. is x = A cos `(omegat+pi/6).` Its speed will be maximum at time ______.
The displacements of two particles executing simple harmonic motion are represented as y1 = 2 sin (10t + θ) and y2 = 3 cos 10t. The phase difference between the velocities of these waves is ______.
A body perform linear simple harmonic motion of amplitude 'A'. At what displacement from the mean position, the potential energy of the body is one fourth of its total energy?
The displacement of a particle of mass 3 g executing simple harmonic motion is given by Y = 3 sin (0.2 t) in SI units. The kinetic energy of the particle at a point which is at a distance equal to `1/3` of its amplitude from its mean position is ______.
A body of mass 0.5 kg travels in a straight line with velocity v = ax3/2 where a = 5 m–1/2s–1. The change in kinetic energy during its displacement from x = 0 to x = 2 m is ______.
Calculate the velocity of a particle performing S.H.M. after 1 second, if its displacement is given by x = `5sin((pit)/3)`m.
For a particle performing circular motion, when is its angular acceleration directed opposite to its angular velocity?
State the expressions for the displacement, velocity and acceleration draw performing linear SHM, starting from the positive extreme position. Hence, their graphs with respect to time.
State the expression for the total energy of SHM in terms of acceleration.
Which one of the following is not a characteristics of SHM?
A particle executing SHM has velocities v1 and v2 when it is at distance x1 and x2 from the centre of the path. Show that the time period is given by `T=2pisqrt((x_2^2-x_1^2)/(v_1^2-v_2^2))`
