Advertisements
Advertisements
Question
An angle is equal to its supplement. Determine its measure.
Solution
Let the supplement of the angle be x°
According the given statement, the required angle is equal to its supplement, therefore, the required angle becomes x°.
Sine both the angles are supplementary, therefore, their sum must be equal to 180°
Or we can say that:
` x + x = 180°`
`2x = 180°`
`x = (180°)/2`
` x = 90°`
Hence, the required angle measures 90°.
APPEARS IN
RELATED QUESTIONS
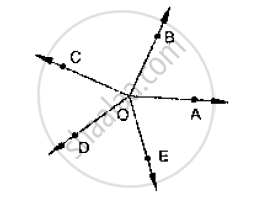
In the given below fig, rays OA, OB, OC, OP and 0E have the common end point O. Show
that ∠AOB + ∠BOC + ∠COD + ∠DOE + ∠EOA = 360°.
In the below fig, POQ is a line. Ray OR is perpendicular to line OS is another ray lying
between rays OP and OR. Prove that ∠ROS = 1 (∠QOS − ∠POS).

Fill in the blank so as to make the following statement true:
If the sum of two adjacent angles is 180°, then the ______ arms of the two angles are
opposite rays
Two straight lines AB and CD cut each other at O. If ∠BOD = 63°, then ∠BOC =
Write the number of endpoints in
(a) a line segment AB
(b) a ray AB
(c) a line AB
How many rays can be drawn through a fixed point O?
Write the complement of `1/2` of a right angle
Write the supplement of 100°
Write the supplement of 0°
Write the supplement of `1/5` of a right angle
