Advertisements
Advertisements
Question
An electric dipole of length 1 cm, which placed with its axis making an angle of 60° with uniform electric field, experience a torque of \[6\sqrt{3} Nm\] . Calculate the potential energy of the dipole if it has charge ±2 nC.
Solution
Torque,
Potential energy,
\[\frac{\tau}{U} = \frac{\left( Ql \right)E\sin\theta}{- \left( Ql \right)E\cos\theta} = - \tan\theta\]
\[ \Rightarrow U = - \frac{\tau}{\tan\theta}\]
\[ \Rightarrow U = - \frac{\tau}{\tan {60}^o}\]
\[ \Rightarrow U = - \frac{6\sqrt{3}}{\sqrt{3}}\]
\[ \Rightarrow U = - 6 J\]
APPEARS IN
RELATED QUESTIONS
(a) Define torque acting on a dipole of dipole moment \[\vec{p}\] placed in a uniform electric field \[\vec{E}\] Express it in the vector from and point out the direction along which it acts.
(c) What would happen if the external field
It is said that the separation between the two charges forming an electric dipole should be small. In comparison to what should this separation be small?
Answer the following question.
Derive an expression for the electric field at any point on the equatorial line of an electric dipole.
A uniform electric field is prevailing in X - direction in certain region. The coordinates of points P, Q, and R are (0, 0), (2, 0) and (0, 2) respectively. Which of the following alternatives is true for the potentials at these points?
A metal sphere of radius 1 cm is given a charge of 3.14 µC. Find the electric intensity at a distance of 1 m from the centre of sphere.
`[epsilon_0 = 8.85 xx 10^-12 "F"//m]`
Electric field on the axis of a small electric dipole at a distance r is E1 and at a distance of 2r on its perpendicular bisector, the electric field is E2. Then the ratio E2: E1 is ______.
The region surrounding a stationary electric dipole has ______
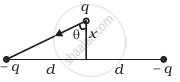
Two charges –q each are fixed separated by distance 2d. A third charge q of mass m placed at the mid-point is displaced slightly by x(x << d) perpendicular to the line joining the two fixed charged as shown in figure. Show that q will perform simple harmonic oscillation of time period.
`T = [(8pi^3 ε_0 md^3)/q^2]^(1/2)`