Advertisements
Advertisements
Question
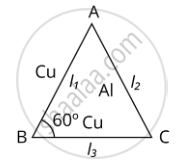
An equilateral triangle ABC is formed by two Cu rods AB and BC and one Al rod. It is heated in such a way that temperature of each rod increases by ∆T. Find change in the angle ABC. [Coeff. of linear expansion for Cu is α1, Coeff. of linear expansion for Al is α2]
Solution
We can solve this by using trigonometry
`cos theta = (1_1^2 + l_3^2 - l_2^2)/(2l_1l_3)`
`2l_1 1_3 = 1_1^2 + l_3^2 - l_2^2`
Now, differentiating both sides:
`2[d(1_1 1_3) xx cos theta + 1_1 1_3 d(cos theta)] = 2l_1dl_1 + 2l_3dl_3 - 2l_2dl_2`
`2[(1_1dl_3 + 1_3dl_1) cos theta - 1_1 1_3 sin theta d theta] = 2(1_1dl_1 + l_3dl_3 - 1_2dl_2)`
`(1_1dl_3 + 1_3dl_1) cos theta - 1_1 1_3 sin theta d theta = 1_1dl_1 + 1_3dl_3 - 1_2dl_2`
`L_t = L_0(1 + αΔt)`
`L_t = L_0 = L_0 αΔt`
`ΔL = Lα xx Δt`
`dl_1 = 1_1α_1 Δt, dl_3 = 1_2α_1Δt`
`dl_2 = 1_2α_2 Δt`
`1_1 = 1_2 = 1_3 = 1`
`dl_1 = 1l_1Δt, dl_3 = 1α_1 Δt`
`dl_2 = 1α_2 Δt` .....(i)
Now, substitute in (i)
`cos theta (1^2 xx α_1 Δt + 1^2α_1 Δt) - 1^2 sin theta d theta = l^2α_1 Δt + l^2 α_1 Δt - 1^2 α_1 Δt`
`2l^2α_1 Δt cos theta - l^2 [sin theta xx d theta]`
= `1^2 [α_1 + α_1 - α_2]Δt`
`1^2 [2α_1 Δ cos 60^circ - sin 60^circ d theta] = 1^2 [α_1 - α_2] Δt`
`2α_1 Δt xx 1/2 - 2α_1 Δt + α_2 Δt = sqrt(3)/2 d theta`
`sqrt(3)/2 d theta = [α_1 - 2α_1 + α_2] Δt`
`d theta = (2(α_2 - α_1)Δt)/sqrt(3)` .....[∵ Δt = ΔT]
`d theta = (2(α_2 - α_1)ΔT)/sqrt(3)`
APPEARS IN
RELATED QUESTIONS
The dimension ML−1T−2 can correspond to
A vertical metal cylinder of radius 2 cm and length 2 m is fixed at the lower end and a load of 100 kg is put on it. Find (a) the stress (b) the strain and (c) the compression of the cylinder. Young modulus of the metal = 2 × 1011 N m−2.
The elastic limit of steel is 8 × 108 N m−2 and its Young modulus 2 × 1011 N m−2. Find the maximum elongation of a half-metre steel wire that can be given without exceeding the elastic limit.
A steel wire and a copper wire of equal length and equal cross-sectional area are joined end to end and the combination is subjected to a tension. Find the ratio of the stresses developed in the two wires .
A steel wire and a copper wire of equal length and equal cross-sectional area are joined end to end and the combination is subjected to a tension. Find the ratio of the strains developed. Y of steel = 2 × 1011N m−2. Y of copper = 1.3 × 10 11 N m−2.
The two wires shown in figure are made of the same material which has a breaking stress of 8 × 108 N m−2. The area of cross section of the upper wire is 0.006 cm2 and that of the lower wire is 0.003 cm2. The mass m1 = 10 kg, m2 = 20 kg and the hanger is light. Find the maximum load that can be put on the hanger without breaking a wire. Which wire will break first if the load is increased?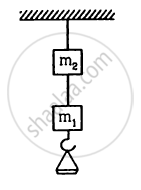
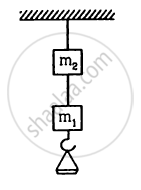
The two wires shown in figure are made of the same material which has a breaking stress of 8 × 108 N m−2. The area of cross section of the upper wire is 0.006 cm2 and that of the lower wire is 0.003 cm2. The mass m1 = 10 kg, m2 = 20 kg and the hanger is light. Repeat the above part if m1 = 10 kg and m2 = 36 kg.
Two persons pull a rope towards themselves. Each person exerts a force of 100 N on the rope. Find the Young modulus of the material of the rope if it extends in length by 1 cm. Original length of the rope = 2 m and the area of cross section = 2 cm2.
A sphere of mass 20 kg is suspended by a metal wire of unstretched length 4 m and diameter 1 mm. When in equilibrium, there is a clear gap of 2 mm between the sphere and the floor. The sphere is gently pushed aside so that the wire makes an angle θ with the vertical and is released. Find the maximum value of θ so that the sphere does not rub the floor. Young modulus of the metal of the wire is 2.0 × 1011 N m−2. Make appropriate approximations.
A wire of length L and radius r is clamped rigidly at one end. When the other end of the wire is pulled by a force f, its length increases by l. Another wire of the same material of length 2L and radius 2r, is pulled by a force 2f. Find the increase in length of this wire.
